This week in week 11 we started Graphing Inequalities. The majority of this is related to our graphing from last year in grade 10 math.

Linear graphs are known as straight line graphs with the equation of y=mx +b.

m is the slope ( )                                                                                                            b is the y intercept

For example: on a graph looks like:

Now when graphing inequalities you have to find a sign that will have a true statement after you have chosen a point on the graph to substitute x and y.

Note that > and < are broken lines on the graph and and  are solid lines like the above graph ( because it is equal to so it includes the line)

• (greater than zero)
• (greater or equal to zero)
• (less than zero)
• (less than or equal to zero)

Now to graph the inequality you have to choose a point on the graph that makes the expression true for example:

to make things easy use (0,0) as the point

•  
•  
• 
• 0 < 6

This statement is true because 0 is smaller than 6. This means that the side that has the coordinate of (0,0) will be shaded in. This will also have a broken line because it is not equal to.

Now when we change the sign to greater to (>) it will flip because 0 would not be greater than 6

 

• 
•  
• 
• 0 > 6
• FALSE STATEMENT

If the sign was or  the line would be solid.

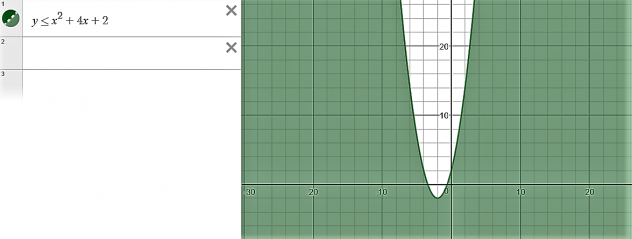
You can also graph a parabola

example:

Step one: Graph the parabola by putting it into standard form.

• 
• 
• 
• 

Graph it from here.

Step two: chose a point on the graph and make a true statement.

• 
• 
• 
• TRUE STATEMENT, 0 is smaller than two

The side with (0,0) as a coordinate will be shaded in and the line will be solid because it is equal to.

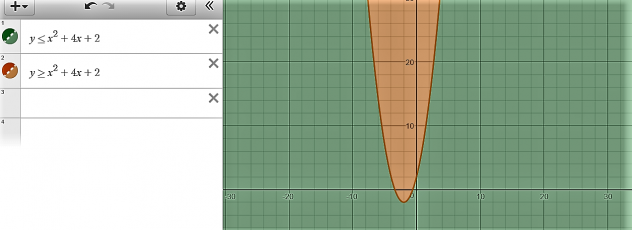
Now if the sign was change the inside of the parabola would be shaded in.

This is how to graph inequalities with linear equations as well as quadratic equations.