Week 10 – Pre-calculus 11

Posted on November 10, 2018 by jessel2016

This week in Math 11 we learned about sign charts which help us find our roots by factoring then once we find our roots we use a number line and our roots to figure out different signs of our parabola and to know which numbers we can use to replace the variable and solve to make the equation true.

Example: 4x $\leq$ 2 $(x^2 - 3)$

This is our equation so, the first thing we do is to factor and find our roots.

4x $\leq$ 2 $(x^2 - 3)$

4x $\leq$ $2x^2 - 6$

0 $\leq$ $2x^2 - 4x - 6$

0 $\leq$ $\frac{2x^2}{2} - \frac{4x}{2} - \frac{6}{2}$

0 $\leq$ $2(x^2 - 2x - 3)$

0 $\leq$ 2(x – 3)(x + 1)

$X_1 = 3$ $X_2 = -1$

Now that we know our roots we can use a sign chart to figure out information about which numbers make the equation true.
We can find out whether our parabola opens up or down.

– – 0 +

(x – 3): x = 3 -1 3

For this one our zero would be 3 because our”x” is equal to zero
on a number line everything to the right of “0” is positive and everything to the left is negative.

– 0 + +

(x + 1): x = -1 -1 3

For this one our zero would be -1 because our “x” is equal to zero

Now if we put these together we can find out to number to make our equation true.

– – 0 +

(x – 3): x = 3 -1 3

– 0 + +

(x + 1): x = -1 -1 3

Now we look at each column of the signs and multiply them

– – 0 +

(x – 3): x = 3 -1 3

– 0 + +

(x + 1): x = -1 -1 3

(-)(-) = + (-)(+) = – (+)(+) = +

Positive(+) Negative(-) Positive(+)

Now if we look back at our original equation: 4x $\leq$ 2 $(x^2 - 3)$

we see that 4x is less than or equal to $ 2 $(x^2 - 3)$
so to figure out which section makes the equation true we would get a number from each section and plug it in to our original equation to see whether it is true or false

– – 0 +

(x – 3): x = 3 -1 3

– 0 + +

(x + 1): x = -1 -1 3

(-)(-) = + (-)(+) = – (+)(+) = +

Positive(+) Negative(-) Positive(+)

Section 1: use -2 Section 2: use 0 Section 3: use 4

NOTE: Always use “0” as a test number if possible.

Section 1: use -2

4x $\leq$ 2 $(x^2 - 3)$

4(-2) $\leq$ 2 $((-2)^2 - 3)$

-8 $\leq$ 2 (4 – 3)

-8 $\leq$ 2 (1)

-8 $\leq$ 2

True

-8 is less than 2

Section 2: use 0

4x $\leq$ 2 $(x^2 - 3)$

4(0) $\leq$ 2 $((0)^2 - 3)$

0 $\leq$ 2 (0 – 3)

0 $\leq$ 2 (-3)

0 $\leq$ -6

False

0 is NOT less than -6

Section 3: use 4

4x $\leq$ 2 $(x^2 - 3)$

4(4) $\leq$ 2 $((4)^2 - 3)$

16 $\leq$ 2 $(16 - 3)$

16 $\leq$ 2 $(13)$

16 $\leq$ 26

True

16 is less than 26

Now that we know which ones are true and which ones are false we can write our answer.

Because our sections that are true are the outer sections we write our answers for “x” separately.
if the true section was the middle section and the outer sections were false we would combine our answer for “x”.
In our original equation we used the “equal to” sign as well , so we would use this for our answer as well.

Answer:

x $\leq$ -1

and

x $\geq$ 3

Week 9 – Precalculus 11

Posted on November 3, 2018 by jessel2016

This week in precalc 11 we learned about how to find maximum values and revenue using quadratic functions, we can also find different variables depending on the word problem for example if there’s a question about area then the question may also ask for the length and width and we have to use the quadratic function to solve for the side lengths along with the maximum values.

Example: A company can sell 56 phones, each one being sold for $300. For every $30 the price increases, the number of phones sold lower by 3. What is the maximum revenue that the company can make by selling the phones?

The formula we use for revenue is: (price)(# of sales)

so knowing this lets see what we know

we have 60 phones
each one is sold for $300
the price can increase by $30
But then the number of phones drops by 3
Were trying to find the maximum value

know we need to use a variable to find out our maximum revenue

The variables will be how many phones we decrease by and how much we increase the price

So lets plug in the number we know into the formula

(300 + 30x)(60 – 3x)

Now we can solve for the variables by completing the square but first we distribute

(300 + 30x)(60 – 3x)

18,000 – 900x + 1,800x – $90x^2$

$-90x^2$ + 600x + 15,000

Now we can complete the square and find our vertex which will give us our “x” value and maximum revenue.

$\frac{-90x^2}{-90}$ + $\frac{900x}{-90}$ + 18,000

-90( $x^2$ – 10 + 25 – 25) + 18,000

-90 $(x - 5)^2$ + 18,000

Vertex is : (5, 18,000)

Our “5” shows our value for “x” and the 18,000 shows our maximum value but because in this question it’s asking for revenue it is our maximum revenue.

Sometimes a question may ask, “which price for a phone will maximise the revenue?”

and what you do is go back to our formula with the numbers

(300 + 30x)(60 – 3x)

and in this case the “(300 + 30x)” talks about the price for a single phone so we plug in our “x” value we got from our vertex and solve to find our the price of one phone that would maximise the revenue.

(300 + 30x)

(300 + 30(5))

(300 + 150) = 450

so this means the price of $450 for one phone will maximise the revenue in this question.

Week 8 – Pre-calculus

Posted on October 27, 2018 by jessel2016

This week in Precalc 11 we learned about graphing quadratic functions and how to analyse them. We do this to see what we know just by looking at the function. Just by looking at a quadratic function we can find the y-intercept, whether the parabola is opening up or down and knowing whether we have a minimum or maximum vertex. That is what we can find when we only see the function but if we see the function graphed we can find a lot more. Along with what we can find with just the function we can find the axis of symmetry, the coordinates of the vertex, the x-intercept(s), the domain and the range.

Image result for parabola

The way we find out all this information just by looking at the function of this graph is.

y= $ax^2 + bx + c$

we see that the parabola is opening up so that would mean that the coefficient of $ax^2$ has to be positive because it is the leading coefficient of the highest term.
The way we find out the y-intercept is that it’s the point on the graph where “x” is zero and the parabola crosses through the y-axis. The point where the parabola crosses is the intercept.
so in our equation if we were to make all of our X’s zero then that would just leave us with “c” which would be our y-intercept.

Image result for parabola

Now if we look at this parabola on a graph we can find out a lot more.

when we tried to find out the y-intercept we knew that the “x” have to be zero and the parabola had to cross the y-axis but now if we want to find the x-intercept we do the opposite. the “y” value must be zero and the parabola must cross the x-axis and the point where it crosses is our x-intercept.
The vertex can be the highest or lowest point on a parabola. The vertex on this graph would have (3, 1) as it’s coordinates. The way we see if it’s a maximum or minimum vertex is whether the parabola is opening up or down. If it opens down it would have a maximum vertex, if it was opening up it would have a minimum vertex.
The axis of symmetry is when you could cut the parabola in half and both sides would be symmetrical. On this graph if we cut vertically on the “3” of the x-axis it would cut the parabola perfectly in half and both sides would be symmetrical, so to show this we say that x=3 for the axis of symmetry.
The domain and range talk about the restrictions that the x and y axis have. Range is for y and domain is for x. In the graph we see that along the x-axis the parabola has negative points and positive points so we would say that “X” is an element of all real numbers.
For the Range we see the parabola never crosses the negative side and is only positive so knowing that we have a minimum vertex and the y for the vertex coordinates is 1 we can say that y is greater or equal to 1.

Week 7 – Precalc 11

Posted on October 22, 2018 by jessel2016

This week in Precalc 11 we learned about discriminant’s. A discriminant is a part of a formula that helps you figure out whether your quadratic formula is going to have no real roots, 1 real root or 2 equal real roots, or if it has 2 unequal real roots. We figure this out by plugging in number into the formula and seeing if it is less than 0, equal to zero, or greater than zero.

The original formula we get the discriminant from is:

We take this formula and only use the $b^2 - 4ac$
To know which numbers go in place or the variables we look at the quadratic equation.

$ax^2 + bx + c = 0$ NOTE: it must always equal zero, this way you know which one is “a” and “b” and “c”. AND MAKE SURE YOU DON”T FORGET YOUR SIGNS.

Example: $5x^2 - 8x + 6 = 0$

so just by looking we know that it equals zero.
and that a=5, b=-8, and c=6
so now that we know these things we can plug them into our discriminant formula.

$b^2 - 4ac$

1) $(-8)^2 - 4(5)(6)$
2) 64 – 120
3) -56 < 0

We see that -56 is less than zero which means they’re no real roots.
If the discriminant was greater than “0” the quadratic would have 2 distinct real roots
If the discriminant was equal to “0” the quadratic would have 1 real root or 2 equal real roots.

Week 6 – Precalc 11

Posted on October 13, 2018 by jessel2016

This week in precalc 11 we learned how to solve quadratic equations by completing the square. This method is used when you cannot factor to find the square. Completing the square allows you to solve for any quadratic equation no matter what it is.

To complete the square we need to use a zero pair.

$x^2 + 4x + 2 = 0$

In this equation we cannot factor because we see that no factors of 2 will add to equal 4.
So instead of factoring we use the first 2 terms to figure out what our zero pair will be.

$x^2$ + 4x + _ – _ + 2 = 0

What we do is make the first two terms and the zero pair a perfect square trinomial
the way we find out the zero pair is.

$(\frac{4}{2})^2$ = 4

we got the 4 from the 4x in the equation
now once we figure out our zero pair we add it back into the equation

$x^2$ + 4x + 4 – 4 + 2 = 0

Then we take the first three terms

$x^2$ + 4x + 4

and we factor this trinomial

(x+2)(x+2) or $(x+2)^2$

then we simplify whats left which is the -4 + 2, and we get.

$(x+2)^2$ – 2 = 0

now we solve by isolating the variable

$(x+2)^2$ – 2 = 0

$(x+2)^2$ = 2

$\sqrt{(x+2)^2}$ = $\pm \sqrt{2}$

x + 2 = $\pm \sqrt{2}$

x = -2 + or – $\sqrt{2}$

we use plus or minus because we can have two values for “x”
and because we don’t have a specific value for “x” and our answer is a square root this means our answer is irrational.

Week 5 – Precalc 11

Posted on October 5, 2018 by jessel2016

This week in precalc I learned how to find the perimeter for squares using by only being given the area. To do this we use radicals and make ourselves and equations and then simplify if we can. Some of these questions are different some ask you to find the perimeter for certain square shapes or blocks. Today i’m just going to talk about solving for only squares.

Finding the perimeter of a square with only area given:

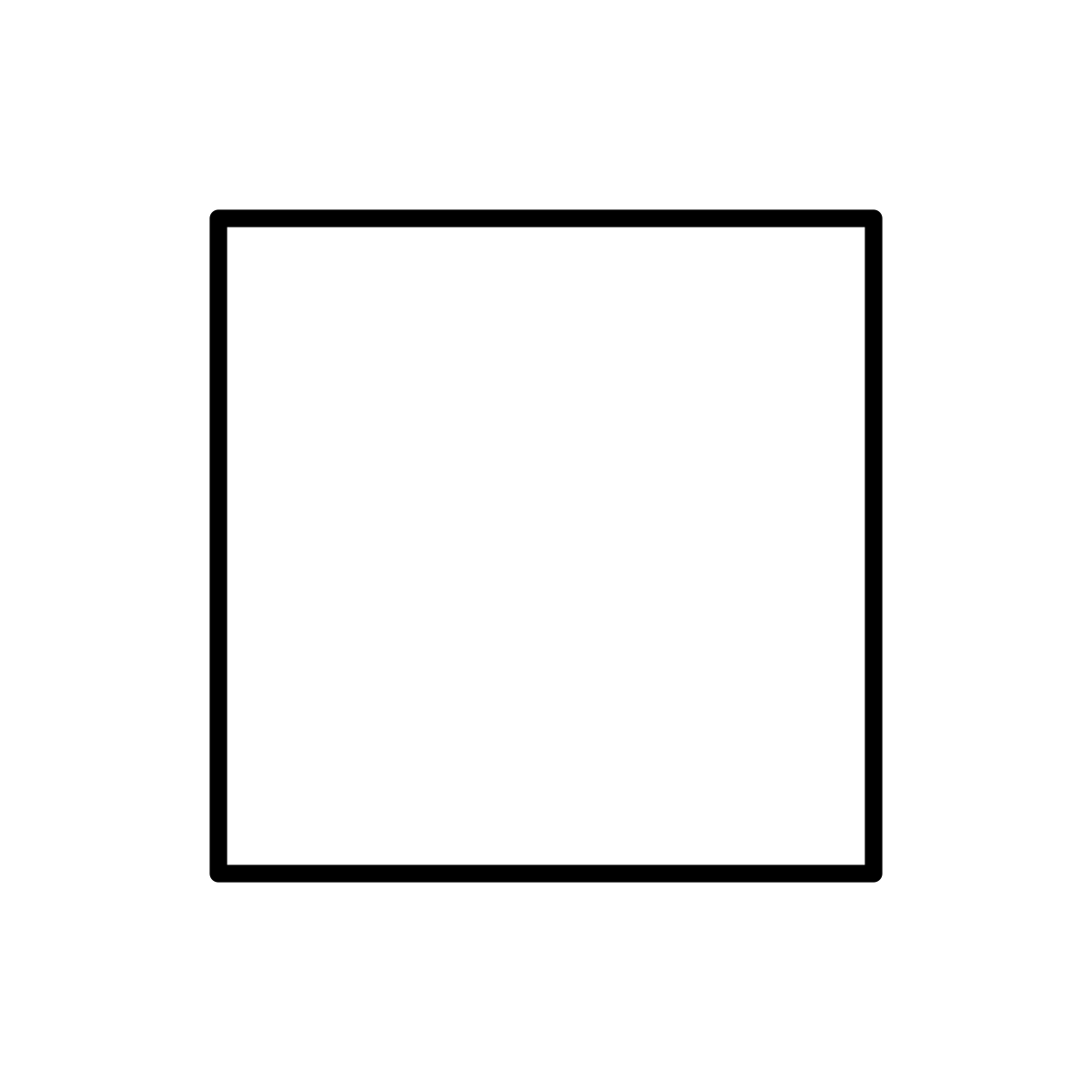Area of this square = 40 $units^2$

Now to find out the perimeter of this square we need to find the lengths

So what do we know?

We know that all sides are the same because it’s a square
we need to add the side lengths to find the perimeter

So how do we find the side lengths?

well we use the formula that we use to find area
- Base x Height = Area (b x h = a)

So if we have the area which is 40 $units^2$ t find the side lengths we square root.

Which means that $\sqrt [2]{40}$ is our side length.

So Now that we know our side lengths we can use the formula; Perimeter = side length + side length + side length + side length. This will help us find our perimeter.

$\sqrt [2]{40}$ + $\sqrt [2]{40}$ + $\sqrt [2]{40}$ + $\sqrt [2]{40}$ = P

Because we are adding radicals we keep the radicand the same and only add the coefficients and for $\sqrt [2]{40}$ the coefficient would be 1 because there is an invisible 1 outside the root.

Answer: $4 \sqrt [2]{40}$

Simplified to: $8 \sqrt [2]{10}$

The Feeling of Fear – English 11

Posted on October 2, 2018 by jessel2016

This image shows fear because this is a photo I took when I was home alone and someone randomly rang my door bell twice and this is what I saw when I looked out the window. The rest of the night I was really cautious around my house, I was hesitant to turn on lights or make noise and overall just scared in my own home. I feel like now I’m just more cautious to answer doors or meet or talk to strangers because I don’t know whats going to happen. I think the only way to reduce the power it has over me is for me to be more optimistic and not think that every situation or interaction I come across is going to be bad. I learned that it’s possible to feel fear even in places that you may feel the most safe. I learned that I need to feel safe around others.

Week 4 – Precalc 11

Posted on September 27, 2018 by jessel2016

This week in Precalc 11 we learned how to add radicals. Adding radicals is a lot like adding polynomials with like terms because the way you tell if it’s a like term is to see if the radicals have the same radicand which is the number under the root sign. but you do not add the radicands you add only the coefficients and keep the radicand the same. In an equation when you simplify all the radicands may not be the same so this means you can have an expression as your answer.

Ex. $2 \sqrt {5} + 5 \sqrt {5} + 3 \sqrt {5}$

In this equation you would only add the coefficients but leave the radicands the same. DO NOT ADD THE RADICANDS!

Answer:

$2 \sqrt {5} + 5 \sqrt {5} + 3 \sqrt {5}$ = $10 \sqrt {5}$

Because 2 + 5 + 3 = 10 and you leave the Radicand $\sqrt {5}$ the same

Now onto bigger equations where you simplify but the answer is an expression:

Ex. $3 \sqrt {18} + 2 \sqrt {24} + \sqrt {50}$

Answer:

$9 \sqrt {2} + 4 \sqrt {6} + 5 \sqrt {2}$

I see that $9 \sqrt {2}$ and $5 \sqrt {2}$ are like terms so I add the coefficients but leave the radicands the same.

$14 \sqrt {2} + 4 \sqrt {6}$ <= this would be my final answer because I can’t simplify anymore.

Always simplify if you can to make it easier and to have the best possible answer.

Why Do People Exclude Others for Not Fitting In?

Posted on September 24, 2018 by jessel2016

In the movie Wonder, directed by Stephen Chbosky, the protagonist August Pullman who was born with Mandibulofacial Dysostosis or Treacher Collins Syndrome, enters fifth grade at a public elementary school for the first time. August is insecure about the way he looks so to hide his face he wears an astronaut helmet but takes it off before he enters the school. Auggie gets made fun of because of his facial condition and struggles to make new friends. One day a boy named Jack Will decides to sit with Auggie at lunch as he sees Auggie is sitting alone. Auggie and Jack begin to bond, start to hangout after school and get to know each other more. During the school’s end year field trip Auggie and Jack go to the forest and run into some older boys from a different school. During this interaction the boys made fun of Auggie’s face and started to push Jack and Auggie around. As Jack and Auggie were being pushed to the ground older kids from Auggie and Jacks school came and began to fight the older kids from the other school so that Jack and Auggie could run away. After the fight was over, Auggie and Jack found the older boys from their school to thank them for what they had done. From that moment many different children began to accept Auggie despite the way he looks. Auggie made friends and people saw him for the person he was and not how he looks. Humans can be accepting; we need to look past what we see on the outside so that we can accept and include people for their more deep and meaningful qualities. This movie shows that even if people are different on the outside we can all accept each other for what’s on the inside, our personalities standout more than our appearances and we don’t need to exclude each other because of the way we look.

Citation:
https://www.scmp.com/culture/film-tv/article/2121745/film-review-wonder-room-star-jacob-tremblay-plays-boy-facial

Week 3 – Precalc 11

Posted on September 20, 2018 by jessel2016

This week in Precalc 11 we learned about infinite geometric series. This means that a geometric series has no ending to it, it has an infinite amount of terms. Which learned about diverging and converging infinite geometric series. It is not possible for us to find a sum for a diverging infinite geometric series, but for converging we can use the formula: $S_n=\frac{a}{1-r}$ n=∞ to determine whether a series is converging or diverging you look at the “r”.

Diverging: r>1 or r<-1

Converging: -1<r<1

Example:

Diverging

3+12+48+192+768…

r= $\frac{12}{3}$ = 4

r= $\frac{48}{12}$ = 4

r=4

Diverging r>1 or r<-1

4>1

for diverging = no sum

Converging

3+2.1+1.47+1.029+0.7203…

r= $\frac{2.1}{3}$ = 0.7

r= $\frac{1.47}{2.1}$ = 0.7

r=0.7

Converging -1<r<1

-1<0.7<1

For converging we use the formula to find the sum

n=∞

$S_n=\frac{a}{1-r}$ $S_n=\frac{3}{1-0.7}$ $S_n=\frac{3}{0.3}$

$S_n$ =10

Jesse's Blog

My Riverside Rapid Digital Portfolio

Category Archives: Grade 11

Week 10 – Pre-calculus 11

Week 9 – Precalculus 11

Week 8 – Pre-calculus

Week 7 – Precalc 11

Week 6 – Precalc 11

Week 5 – Precalc 11

The Feeling of Fear – English 11

Week 4 – Precalc 11

Why Do People Exclude Others for Not Fitting In?

Week 3 – Precalc 11