This weeks goal is to teach you how to solve an angle and side lengths in the Trigonometry Unit.
Vocabulary:
SOH: S =  or Sine =
or Sine = 
CAH: C = 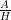 or Cosine =
or Cosine = 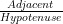
TOA: = 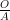 or Tangent =
or Tangent = 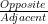
i) Question One: What is the Angle of JEP to the nearest degree? (How to find an angle knowing two side lengths)
 Step 1: Label the triangle
Step 1: Label the triangle
*According to the reference angle
- Across from the reference angle is called the opposite side (O)
- Across from the
 angle is the hypotenuse (H)
angle is the hypotenuse (H)
- And the left over side is the adjacent side (A)
 Step 2: Determine which ratio to use
Step 2: Determine which ratio to use
Use the clues from the triangle. We will be using Cosine because we have the clues from the Adjacent and hypotenuse sides. (CAH)

Step 3: Write the equation
The cos angle is unknown so we will be writing it like :

Step 4: Get the variable by itself/Solve
*when moving the COS to the other side of the equation it becomes 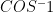

ii) Question two: What is the side length of AP to the nearest degree. (How to find the side length of a triangle knowing one side length and one angle.)

Step 1: Label the Triangle
*According to the reference angle
Step 2: Determine the ratio
Use the clues from the triangle. We will be using Tangent because we have the clues from the adjacent and opposite sides. (TOA)
Step 3: Write the equation/Solve
*We don’t want the variable on the bottom. Reciprocate the fraction and what you do to one side you do to the other.



X= 50cm
iii) Question Three: What is the side length of AK to the nearest degree. (How to find the side length knowing one side length and one angle .)

Step 1: Label the Triangle
*According to the reference angle

Step 2: Determine the ratio
Use the clues from the triangle. We will be using Sine because we have the clues from the hypotenuse and opposite sides. (SOH)
Step 3: Write the equation/Solve

*We don’t want the variable on the bottom. Reciprocate the fraction and what you do to one side you do to the other.



X= 51 cm
+ 8x +12
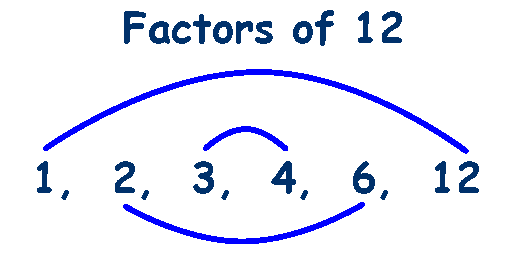
so the x’s will go at the front of the brackets. (x___)(x___)



















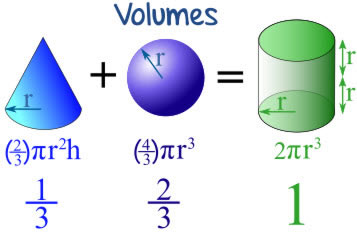 One cone with + One Sphere = The volume of a cylinder
One cone with + One Sphere = The volume of a cylinder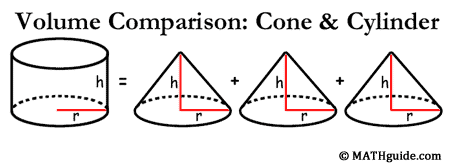 Three cones = the volume of a cylinder
Three cones = the volume of a cylinder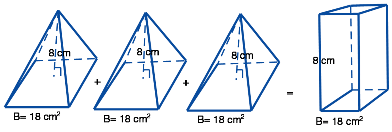 Three pyramids = A Square/rectangle
Three pyramids = A Square/rectangle