In this week we learned how to balance trinomials, to do this we use this formula,

so lets say we have this as our equation,

first we must identify A, B and C,
is A
is B
is C

So using these values we must replace them in the spots of the formula with the matching letters,

Now we will evaluate the equation,

then,

after you get to this point we must find a common factor to divide everything with,

Giving us,

simplified again,

and that’s the final answer!

This week we learned stuff along the lines of  CDPEU and other stuff to help us simplify binomials and trinomials.

for example,

lets say I have something that looks like this,

this binomial would look somehing like this once it is simplyfied

Now, what time what makes 16,

So it would look like this

once you combine all of these you get,

To find the simplest form of we follow these steps…

First,   make two of the expressions because it was squared.

then we will start by multiplying each number by the other

once done all this combine like factors

then we will get rid of any hole numbers

and we will end up with…

PS.  I don’t know the code for the square root symbol so I just used (/) as a replacement.

in this week we of math we learned about radicals and to sum up what a radical is it basically turns a negative into a positive.

so |-5| becomes 5

and something like |-8+5| would be |-3| which makes it 3

but if its -|-5| it becomes -5.

To find a term in a geometric sequence you must use the formula

= a

to give an example here is my pattern  -3, 9, -27, 81…
to find   follow these steps….

First,  becomes 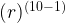 wich is  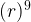leaving you with…

= a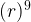

Then you want to add in (a), replace (a) with -3 because it’s your first term. making your equation look like…

= -3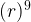

once you have used all the given numbers you have. find the common ratio (r). if you follow the pattern of the sequence you will notice it is being multiplied by (-3) each time. so replace (r) with (-3)

= -3

is -19683

-3 x -19683 is 59,049

therefore = 59,049

–
to find the Geometric series you need to use this formula…

=

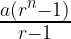
using the pattern above we will find the series of 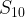 . first lets add in the values we were given straight of the bat. replace (a) with -3, and replace (n) with 10…

=

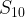if you remember, above we found out that the common ratio was -3, so replace (r) with -3…

=

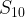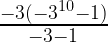
first, we will do wich is,  59,049. then subtract 1 from this giving you, 59,048. now add this in…

=

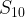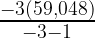
after that multiply 59,048 by -3 giving you, -177,144. now add this to your equation…

=

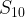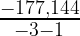
now we must solve the denominator, -3-1 = -4.  your equation should look like this…

=

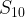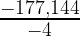
now divide these…

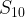= 44,286