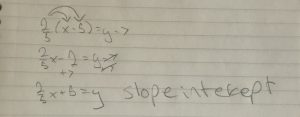this week in math 10 we started our unit on “systems of linear relations” I learned that a solution is the point on a graph at which two lines meet. depending on the relation of the two lines, there can be 1 system, infinite systems, or no systems at all. there is one system if the slopes to not match, there are an infinite amount if the slope and y-intercept of the lines match, and there are 0 solutions if the slopes are the same because this would represent parallel lines.

to find the solution of two lines we can use one of two methods, we can use the elimination method or the substitution method.

do demonstrate substitution for example we have the lines

8x-3y=10 , x-y=5

start off by choosing an equation of the two to isolate either y or x.

x-y=5 now turns into x=y+5

now we take the other equation 8x-3y=10, and replace x with y+5, becomes 8(y+5)-3y=10 , distribute the 8 into y and 5

8y+40-3y=10, combine like terms and isolate the variable to find the y coordinate.

8(y+5)-3y=10

8y+40-3y=10

5y=-30

5y/5=-30/5

y= -6

now to find the x-intercept we go back to one of the original equations and replace all of the y’s with -6

x-y=5

x-6=5

isolate the variable

x=-1 now we have the solution of these two lines as (-1,-6)

no verify this, we input the x and y into both original lines and if they are both equal then the question is complete

now to use elimination, a much quicker method.

we have the lines 2x+3y=7, 5x-3y=28

we can either add or subtract the two equations, the goal is to get two of the terms to cancel out to make the question simpler

2x+3y=7

5x-3y=28

add the equations together, in this case, +3y and -3y are opposites so they can cancel out and we are left with

7x=35 now isolate x

so x=5. now we input 5 into one of the original equations, replacing all of the x’s, to find the y-coordinate. then verify by inputting x and y into both original equations as we did for the substitution method.

group inquiry- short story, poetry- notes-154lep1

group inquiry- english 10-r5tlbf

core competencies – photography 10

this week in math 10 I learned about parallel lines and perpendicular lines. I learned that the slope of parallel lines will always be the same, and that the slope of perpendicular lines will be negative reciprocals. a negative reciprocal for example: if one slope is then the slope of the line perpendicular will be .

I also learned how to convert to point- slope form, to slope- intercept form, to general form, starting with a set of coordinates and a slope.

point- slope : m(x-x2)=y-y2

so if we have a slope of 2/5 and coordinates (5,7) , it would be written out as 2/5(x-5)=y-7

because m= 2/5, x2= 5 and y2= 7

then from there, to convert to slope- intercept form (y=mx+b) we need to isolate y, starting by distributing 2/5 into x and -5, then adding 7 to each side, finishing with +5=y

now to convert to general form (x+y+#=0)  we need to get rid of all the fractions, and make sure the leading coefficient of x is positive.

because 2/5x is already positive we just need to move y over by subtracting it from each side, ending up with -y+5=0. then to get rid of the fractions we multiply everything with the common denominator which in this case is 5. general form: 2x-5y+25=0

https://sd43bcca-my.sharepoint.com/personal/132-mkosola_sd43_bc_ca/Documents/english%2010/literature%20circle%205.docx?web=1

https://sd43bcca-my.sharepoint.com/personal/132-mkosola_sd43_bc_ca/Documents/english%2010/literature%20circle%20reflection%202.docx?web=1

https://sd43bcca-my.sharepoint.com/personal/132-mkosola_sd43_bc_ca/Documents/english%2010/literature%20circle%204.docx?web=1

https://sd43bcca-my.sharepoint.com/personal/132-mkosola_sd43_bc_ca/Documents/english%2010/literature%20circle%203.docx?web=1