November | 2016 | Krista's Blog

${Everything}$ ${I}$ ${Know}$ ${About}$ ${Exponents}$

1.Representing repeated multiplication with exponents-multiplication is the same thing as repeated addition. And exponents are the same thing as repeated multiplication.

Ex. x+x+x= $x^3$

x is the base and 3 is the exponent or how many times x is multiplied by itself .

2.Discribe how powers represent repeated multiplication- powers represent repeated multiplication because you don’t multiply the exponent by the base, you multiply the base with itself, depending on what number the exponent is.

Ex. $5^4$ = $5\times5\times5\times5$

3.Demonstrate the difference between two given powers in which the exponent and the base are interchanged by building models of a given power. The first model is $2^3$ because there are 3 edges that equal 2. the second model is $3^2$ because the length is 3 and the width is 3.

$2^3$

$3^2$

4. Demonstrate the difference between two given powers in which the exponent and the base are interchanged by using repeated multiplication

It is the difference between $2^3$ and $3^2$ the answer is different because when you put $2^3$ , it equals $2\times2\times2$ , but when you write $3^2$ , it equals $3\times3\times3$

5.Evaluate powers with intergral bases (excluding base 0) and whole number exponents.

$(-3)^2$ =9

$(-2)^2$ =4

$(-1)^2$ =1

$1^2$ =1

$2^2$ =4

$3^2$ =9

6.Explain the role of parentheses in powers by evaluating a given set of powers.

${-2}^4$ is the same thing as $({-2}^4)$ because it all equals to ${-1}\times\times2\times2\times2\times2$ . But, ${(-2)}^4$ is equal to ${-2}\times{-2}\times{-2}\times{-2}$

7. Explain the exponent laws for multiplying and dividing powers with the same base.

Product law: 1. Keep the base 2. add the exponents 3. multiply the coefficients

Ex. $(3)4^3\times (2)4^2$ = Keeping the 4 as 4. Adding the exponents (2+3=5). and multiplying the coefficients. (3×2) so you end up with $(6)4^5$

Quotient Law: 1.Keep the base 2. divide coefficients 3. subtract exponents

$5^6\div5^5$ You just subtract the exponents so then it equals 5.

8.Power law=1. Keep the base 2. multiply the exponents 3. don’t forget you coefficants.

$(2^3)^3$

Multiply $3\times 3$

= $2^9$

9. Explain the law for powers with an exponent of 0.

Any base to the power of 0=1, with the exception of 0 as a base, which is undefined.

$5^0$ =1

$3^0$ =1

${5564}^0$ =1

10.Use patterns the show that a power with an exponent of zero is equal to one.

$2^3$ =8

$2^2$ =4

$2^1$ =2

$2^0$ =1

As the exponents decrease by one, the answer is divided by the base.

11.Explain the law for powers with negative exponents

Reciprocal the base(flip) 2. make exponent positive(because of the flip)

$5^{-2}$

= $\frac{1}{5^2}$

12.use patterns to explain the negative exponent law

= $\frac{1}{10^2}$ = $5^{-2}$

$\frac{1}{5^1}$ = $5^{-1}$

13.I can apply the exponent laws to powers to both integral and variable bases

The exponent law applies to bases if there integral or a variable, but they have to be the same base for the laws to work.

Intergral-Multiplication laws

$5^2$ = 5×5

Divison Laws

$5^3\div5^1$ = $5^2$

Power laws

$(5^2)^2$

$5^4$

Variable-Multiplication laws

$x^2$ = $x\times{x}$

Division laws

$x^4\div{x^2}$ = $x^2$

Power Laws

$(x^4)^2$

= $x^8$

For all multiplication laws ${with}$ ${the}$ ${same}$ ${base}$ you add the exponent and keep the base.

For all division laws ${with}$ ${the}$ ${same}$ ${base}$ you subtract the exponents, and keep the base.

For Power laws- You keep the base, and multiply the exponents

(these do not include coefficients)(They also don’t include undefined numbers)

14. I can identify the error in a simplification of an expression involving powers.

Ex. $5^3\times5^2$ =25

The error that was made here is they correctly added the exponents, but then made the mistake of saying that $5^5$ =25

The correct way of doing this is

$5^3\times5^2$

3+2 (adding the exponents)

= $5^5$

= $5\times5\times5\times5\times5$

= $3125$

15.Use the order of operations on expressions with powers-BEDMAS

$(5\times 2^3)$ +4 $\div 4-2$

↓ ↓

= $(5\times8)$ +1-2

↓

=40+1-2

↓

=41-2

= $39$

16. Determine the sum and difference of two powers

Evaluate the individual powers, then add or subtract them

$4^2$ + $3^2$

= 16+9

=25

$6^2$ – $3^0$

=36-1

= $35$

17.Identify the error in applying the order of operations in an incorrect solution

Incorrect Solution

$2^2+4^2$ $\div2-2^2$

↓ ↓ ↓

=4+16 $\div2-4$

↓ ↓

=20 $\div(-2)$

↓

$=-10$

In line 2, the problem was that addition and subtraction was done before division

Correct solution

$2^2+4^2$ $\div2-2^2$

↓ ↓ ↓

=4+16 $\div2-4$

↓

=4+8-4

↓

=12-4

$=8$

As you can see, messing up 1 step effects your whole equation.

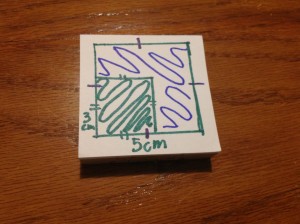
18. Use powers to solve problems (measurement problems)

Find the surface area of the blue shaded square

$5^2-3^2$

=25-9

=16

19.Use powers to solve problems (growth problems)

Your making dough with (magic) yeast in it. every hour, the weight of the dough doubles. The dough weighs 1.2lbs. at the start. What happens to the weight of the dough after

2 hours=1.2∙2=2.4lbs.

2.5 hours=1.2∙2.5=3lbs.

3 hours=1.2∙3=2.6lbs

x hours=1.2∙x=1.2xlbs.

Example, In Science class the students made expanding elephant toothpaste and every 10 minutes the size of the goo doubled. They started out with 5ml of toothpaste, how much toothpaste was there after…

A) 10 minutes: $5 \cdot 2$ = 10ml