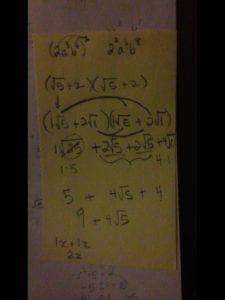This week, I had trouble creating and knowing how to make the “x” and “y” table of values from an equation, and knowing how to graph it.

If you had y = 2x^2 + 8x + 6 you would begin by finding the vertex. You would do that by factoring out 2 from the equation (because that is the value of the first number). So the equation would now become y = 2 (x^2 + 4x + 3) The next step would be to take the 4 divide it by two and then square it. Then, you would take that number (4) and create a zero pair after the 4x in the equation. The equation would now becomre y = 2 (x^2 + 4x + 4 – 4 +3). Then you put brackets around the first three numbers of the equation, y = 2 (x^2 + 4x + 4)(-4 +3). After this, you would go back to the number that you had to divide by two and then square (4), and root it, which gives you two. So the equation would now become, 2 (x + 4) (x + 4 ) (-1), which would become 2 (x + 4)^2 (-1). This would give you your vertex of (-4, -1). Once you have found your vertex, you are able to put it into the middle of the table, and move up by two, and down by two. The top number on the “y” values of your table will be the same on the top and bottom. These will give you your points for your graph. Once you have these you will be able to plot them on the graph, which you can draw based on where you points on (move the graph higher or lower). That is how you find the vertex, points, and graph an equation.

For this chapter in math, I have struggled. However, I learned a trick that can help make long factoring questions faster and more clear.

For these types of questions, you can substitute what’s in the brackets on both sides for a variable. If they are both the same then you can make the the same variable (a) and if they are both different you can make them different variables (a and b). After, you re-write the equation with the variables in place of what was in the brackets. After this, you can factor it. In this equation, the root of 49 is 7, so you write 7 on both sides of the equation, since the variables are squared you put an “a” next to the 7. Next, you root 9, which is three. Since three is negative, one three on a side will be negative, and the other three will be positive in the other brackets. You would do that same as the “a” and put the “b” variable next to both threes. Once youo do this, you re-write the equation replacing what the variables where for what was originally in the brackets. After, you factor what your equation is. You would factor the number that is in front of the brackets to the brackets in front of it. After you so this, you are left with your answer.

This week in math I have had some trouble figuring out some of the different strategies for factoring. I will explain how to factor using the completing the square technique.

For this example, the first thing you do is add a zero pair at the end f the equation but before the equal sign, in this equation that was +16 and – 16. Then, you take take the first three terms as your equation to solve first. To do this, you take the second term, and half it, and then square it, in this equation that was 8. Next you take one of the answer you get from that, half all of the equation (only the first three terms), and write them out next to each other (which can be simplified by writing it once and squaring it), adding on the rest of the equation that we where ignoring before. Next, you want to isolate x. To do this, you first have to take away what is not in the brackets first. In this equation, that is -6 (you would add +6 to both sides of the equation). Next, you have to get rid of the square, and to do that youo would root both numbers of both sides of the equation. When you root the other side of the equation, it becomes +-. Since the square and brackets are now gone, you can isolate the x. In this equation, you moved the four to the other side of the equation by adding -4 on both sides. This, would give you the answer.

This week, I was away from a lesson we did. After asking a few people, none seemed to have any notes. One of the many things that I learned this week, is that you can ask Mrs. Burton for the notes, if you can’t find any. Because of this, I was very confused for this lesson on how to do anything. One of the things I was most confused with though, was solving an equation. To better understand how, the “gift” technique.

For this equation, the seven is in front of the root. This can be thought of as a bow on top of a gift, you need to take it off before you can “open” any of the rest of the equation. To do this, you have to divide both sides by seven to get rid of it. Next, you have to get rid of the root or the “wrappping”. To do this, you have to square both sides. After you have done this, you have one last step to get to the “gift” (to isolate x). You have to get rid of the number that is next to the x. To do this, you divide both sides by two (or the number that is there). And this will give you your gift, or your answer.

This week, I’ve had a lot of trouble with the math. I’m stilll trying to uderstand how to do each question, but I have figured out a mistake that I need to work on correcting and understanding. I need to remember to simplify my equations after I have written them out (completing the BEDMAS part of the question).

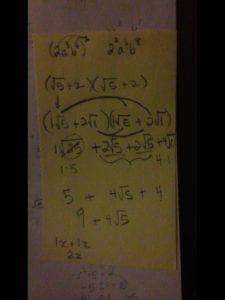
To be able to simplify these equations, a tip I learned is to put a 1 in front of each root (unless there is already a number there) in order to make simplifying and combining like terms more clear. After having done this, you can begin simplifying and combining the question. In the picture above, 1 root 25 became root 5, because 5 x 5 is 25, times 1 (because of the number you put in front of the root), which is five. For root four, you multiply the root by the number in front of the root (1) by the number in the root (4), to get 4. When combining two like terms, the number inside the root (radical) stays the same, however, you do multiply the numbers outside the root with each other.