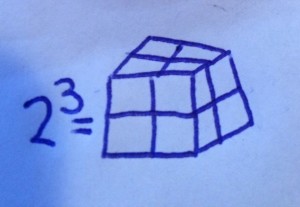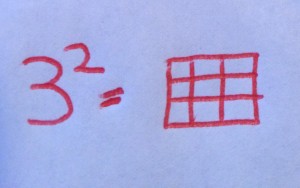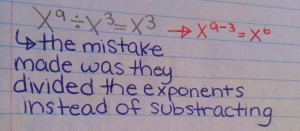ExponentsHUBBARD2016 – Ashiana's Blog

1. Represent repeated multiplication with exponents

You can take repeated multiplication and turn it into an exponent by counting the number of times you multiply a number by itself which becomes the base and use the number of times as the exponent.

Ex: $5 \cdot 5\cdot 5\cdot 5$ would be $5^4$ because there are 4 5’s.

2. Describe how powers represent repeated multiplication

Powers represent repeated multiplication in the exact opposite way the number of the exponent is the amount of times you multiply the base number by itself.

Ex: $3^6$ would be $3\cdot 3\cdot 3\cdot 3\cdot 3\cdot 3$ because 6 is the exponent so there are 6 3’s.

3. Demonstrate the difference between the exponent and the base by building models of a given power, such as $2^3$ and $3^2$

The base is equal to the amount of side lengths of the model and the exponent is the amount of sides the model has.

Therefore $2^3$ as a model is:

and $3^2$ as a model is:

4. Demonstrate the difference between two given powers in which the exponent and the base are interchanged by using repeated multiplication, such as $2^3$ and $3^2$ .

The difference in the powers $2^3$ and $3^2$ is in the first power $2^3$ , 2 is the base and 3 is the exponent, therefore 2 is the number you are multiplying by itself 3 times because 3 is the exponent so it would be $2\cdot 2\cdot 2$ which is equal to $8$ Whereas in the second power $3^2$ , 3 is the base and 2 is the exponent, therefore it would be $3\cdot 3$ which is equal to $9$

5. Evaluate powers with integral bases (excluding base 0) and whole number exponents.

Evaluating powers with integral basses and whole number exponents works by using repeated multiplication.

$\langle {-3} \rangle^2$ → ${-3}\cdot {-3}$ = $9$

$\langle {-2} \rangle^2$ → ${-2}\cdot {-2}$ = $4$

$\langle {-1} \rangle^2$ → ${-1}\cdot {-1}$ = $1$

$1^2$ → $1\cdot 1$ = $1$

$2^2$ → $2\cdot 2$ = $4$

$3^2$ → $3\cdot 3$ = $9$

6. Explain the role of parentheses in powers by evaluating a given set of powers such as $\langle -2\rangle ^4$ , $\langle -2^4 \rangle$ and $-2^4$

When the exponent is $\langle -2\rangle ^4$ it is ${-2} \cdot {-2} \cdot {-2} \cdot {-2}$ which is equal to ${+16}$ , because 4 is an equal number of negatives, this is because the – and the 2 are together in parenthesis and the exponent is outside of both of them. $\langle -2^4 \rangle$ $-2^4$ are the same you do BEDMAS because it is exponent first you go $2^4$ first which is equal to ${+16}$ and then you multiply 16 by it’s coefficient which is -1. Therefore $-1\cdot 16$ is equal to ${-16}$

7. Explain the exponent laws for multiplying and dividing powers with the same base.

The law for multiplying powers with the same base is keeping the base adding the exponents.

$2^6$ • $2^3$
$2^{6+3}$
$2^9$

With a coefficient you would multiply the coefficients by each other.

( $5$ ) $2^6$ • ( $6$ ) $2^3$
$2^{6+3}$ and $5 \cdot 6$
( $30$ ) $2^9$

The law for dividing powers with the same base is subtracting the exponents.

$2^6$ ÷ $2^3$
$2^{6-3}$ or could be demonstrated with repeated multiplication $\frac{2\cdot 2 \cdot 2\cdot 2 \cdot 2\cdot 2} {2 \cdot 2 \cdot 2}$
then you would eliminate the corresponding and be left with $2^3$

With coefficients you would go:

( $30$ ) $2^6$ ÷ ( $5$ ) $2^3$
$2^{6-3}$ or could be demonstrated with repeated multiplication $\frac{2\cdot 2 \cdot 2\cdot 2 \cdot 2\cdot 2} {2 \cdot 2 \cdot 2}$ and then you go $\frac {30}{5}$
then you would eliminate the corresponding and be left with ( $6$ ) $2^3$

8. Explain the exponent laws for raising a product and quotient to an exponent.

When raising a product or quotient to an exponent you use the power law, the rules for the power law are to keep the base multiply the exponents.

Example of power law:

$\langle 3^2 \rangle ^5$ → $3^{2 \cdot 5}$ → $3^{10}$ = $59049$

9. Explain the law for powers with an exponent of zero.

Any power with the exponent of 0 is equal to 1 unless the base is equal to 0.

This is because $\frac {3^2}{3^2}$ when doing BEDMAS becomes $\frac {9}{9}$ which is equal to 1. So when using the quotient law $3^2$ – $3^2$ is equal to $3^0$ which makes it equal to 1.

10. Use patterns to show that a power with an exponent of zero is equal to one.

A pattern of powers with the same base demonstrate that when dividing the answer by the exponent you will get 1 when it comes down to zero as the exponent

$2^8$ = $256$

$2^7$ = $128$

$2^6$ = $64$

$2^5$ = $32$

$2^4$ = $16$

$2^3$ = $8$

$2^2$ = $4$

$2^1$ = $2$

$2^0$ = $1$

Each time you go down you divide by the base number so in the case 2 so when it comes to zero the answer is 1.

11. Explain the law for powers with negative exponents.

Any base except zero raised to a negative exponent equals the reciprocal of the exponent so that it becomes positive.

ex: $2^{-3}$ becomes $\frac {1}{2^3}$ which equals $\frac {1}{8}$

12. Use patterns to explain the negative exponent law

When the exponent in the power is negative you must switch it to it’s reciprocal so that the answer can be positive. If the bases are the same the pattern will be divide every answer by 2 to get the one down.

$2^{-1}$ = $\frac {1}{2^1}$ = $\frac {1}{2}$

$2^{-2}$ = $\frac {1}{2^2}$ = $\frac {1}{4}$

$2^{-3}$ = $\frac {1}{2^3}$ = $\frac {1}{8}$

$2^{-4}$ = $\frac {1}{2^4}$ = $\frac {1}{16}$

$2^{-5}$ = $\frac {1}{2^5}$ = $\frac {1}{32}$

$2^{-6}$ = $\frac {1}{2^6}$ = $\frac {1}{64}$

$2^{-7}$ = $\frac {1}{2^7}$ = $\frac {1}{128}$

$2^{-8}$ = $\frac {1}{2^8}$ = $\frac {1}{256}$

$2^{-9}$ = $\frac {1}{2^9}$ = $\frac {1}{512}$

13. I can apply the exponent laws to powers with both integral and variable bases.

The exponent laws can be applied to both integral and a variable bases because the laws work on the exponent not concerning the base as long as the bases are the same.

Ex: $2^3$ • $2^6$ $2^{3+6}$ = $2^9$

With a variable: $x^3$ • $x^6$ $x^{3+6}$ = $x^9$

14. I can identify the error in a simplification of an expression involving powers.

Error’s made in evaluating powers are either because of the product law, quotient law, power law, zero law, or negative exponents.

Here are examples of me correcting errors in expressions involving powers:

Example of power law:

Example of quotient law:

Example of product law:

Example of zero law:

Negative exponent:

15) Use the order of operations on expressions with powers.

Using order of operations is using BEDMAS. You use BEDMAS on expressions with powers when the bases of multiplication/division expressions aren’t the same or when you are adding and subtracting powers no matter what the base.

Example of different base mistake and correction:

fullsizerender-6

Example of adding powers:

fullsizerender-14

Example of subtracting powers:

fullsizerender-15

16) Determine the sum and difference of two powers.

There is no law for finding them sum of two powers you must simply use BEDMAS so you evaluate the exponents first and then add or subtract them for your answer. Therefore to solve this equation you have to do the exponents first and then add them together.

$3^2$ + $4^2$

$9$ + $16$

$25$

17) Identify the error in applying the order of operations in an incorrect solution.

Order of operations is BEDMAS, Brackets, Exponents, Division &Multiplication {in the other they happen} Addition & Subtraction {in the order they happen.} However if any of the laws apply you must do those first.

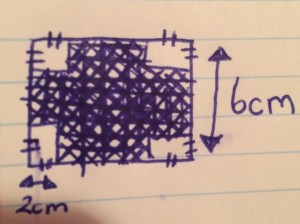
18) Use powers to solve problems (measurement problems)

To find the area the blue part of the square you have to go $6^2$ ,because 6cm is the measurement of the side lengths of the full square, minus (4) $2^2$ because there are 4 mini squares in white with equal side lengths of 2cm.

$6^2$ – (4) $2^2$

= $36$ -16

= 20

To find the volume of the cube you have to go $4^3$ because a cube is made up of three equal sides.

$4^3$ = 64

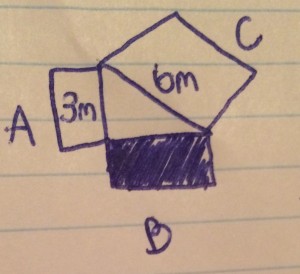
To find the length of a triangle with squares you have to use the pythagorean theory which is $a^2$ + $b^2$ = $c^2$ . But because this figure is missing angle B not angle C you have to use subtraction and go $c^2$ – $a^2$ = $b^2$ .

= $6^2$ – $3^2$

= 36m – 9m

=25m

But 25m is the area of the square however we are looking for the side length so we have to find the square root of 25 which can either be 5 or -5 but because this is a figure it has to be greater than 0 and only 5 is therefore the answer is 5m.

The final measurement I will demonstrate is finding the are of the blue part of the figure down below showing a circle inscribed in a square meaning it’s touching the edges of the square perfectly. The whole measurement of the figures side lengths is 13 therefore it would be $13^2$ minus pi multiplied by the radius of the circle squared which would be ${6.5}^2$ because the radius is equal to half the diameter which was 13.

= $13^2$ – π ( ${6.5}^2$ )

= 169 – π (42.25)

= 169 – 132.73

= 36.27 $cm^2$

19) Use powers to solve problems (growth problems)

My pet hamsters weight triples once a month. It weighs 20 pounds now how much will it way in:

a) 2 months b)4 months c) 6 months

a) 20 x $3^2$

b) 20 x $3^4$

c) 20 x $3^6$

Growth problems can be solved by using exponents because in this example because my hamster started at 20 pounds and is tripling every month so at 2 months it becomes 20 x $3^2$ 20 for the amount of pounds, 3 for the tripling and to the power of 2 because it has been 2 months of tripling which means it has to be 20 x 3 x 3. Therefore with any amount of months it would be 20 x $3^x$

20) Applying the order of operations on expressions with powers involving negative exponents and variable bases.

$\frac {\langle a^{-5} b \rangle ^{-3}}{\langle a^4 b \rangle ^{-2}}$

When you are applying the order of operations on expressions with powers involving negative exponents and variable bases the first thing with this question you do is the power law.