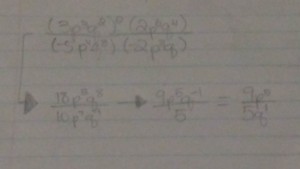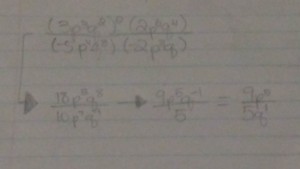Article Reflections

Article 1: Fake Facebook Accounts

This article doesn’t really affect me because I don’t have any social media accounts, so I wouldn’t be effected by the problem. But I can see how it can effect other people and how it could become dangerous. If one person is able to make one fake account, surely they would be able to make many. It allows the person to be able to manipulate the thoughts of others by making it seem that many people agree with the opinion. In our society, many people agree with majority because they don’t want to be on the side that looses or be different and be judged by other people. So if you were to create a post with your opinion and use the other fake accounts to ‘agree’ with your opinion, other people might start thinking that it’s a good idea. In this case, the posts are targeting Farrell. Fake accounts also need to steal images from the internet to make their profile seem real, so people will start thinking that it’s okay be a fake person but end up stealing images of real people to make their account ‘come to life’. I think that having fake account is also tied with impersonating someone on social media, which is also illegal. Many people think that it’s okay to impersonate their favourite celebrities or youtubers online, but in reality it’s actually illegal. So I think that in this world, people find it okay to be someone that they really aren’t and with technology today, many people can’t tell the difference between real and fake accounts.

Article 2: Fake “PLEASE REPOST SO I CAN FIND MY FAMILY!!!” Posts

Again, since I don’t have social media, I am unable to relate to this but I find it to be over dramatic. I think that people shouldn’t go to social media to find their family because people will end up thinking that it’s just for attention. Since there are so many posts like that circling around, it’s easy for people to just assume that they are all fake. I also think that for people who actually have missing family members don’t appreciate people who are faking it and making it seem like a big thing. If I were to have my little brother missing and then find all of these fake missing family posts, it would feel hurtful to see people whom are taking the situation lightly. I find most chain posts to be ridiculous as they’d be like “Repost or else spiders will invade your bedroom tonight”. I think that if you were to go online, most things that tell you to repost are ridiculous and are probably not worth it. Yet some people find it to be a big thing. So all the meaning less reposts probably outweigh the things that people repost to gain awareness. But at the same time, do you really want to take your problems to social media and have the whole world see it? Is it really worth it at the end?

Data Analysis Thoughts

I think that in our society, we are unable to realize that two questions could mean the exact same meaning while being worded differently. We look at names with more power as a more reliable source when there could always be a bias without us knowing. I think that statistics in our society today is to for people to prove their thoughts and ideas to others. It could be used for advertising for some new product or even for people’s mathematical capability. Statistics are able to show how people are able to be convinced to do something, just because of majority. They are able to be used to persuade people into doing things without them thinking that it’s a bad thing. They are also used in scientific ways to test out new medications and different experiments.
Statistics can be used to show many different things in our society. They are used for both advertising, competition and scientific matters. The questions you could answer in a poll all depend on how the author writes the questions. It’s able to change your thoughts from positive to negative and vice versa. I also learned that many people don’t understand statistics to a full extent. Some think that the percentage that they see is from a larger group of people when in reality, it’s only from 10-100 people who were surveyed.
Lots of people aren’t able to tell that some statistics are to try to make you change your thoughts on something. There are also many inaccurate statistics that are mathematically incorrect and aren’t true. Like in the last question, some people sometimes think that the percentage that they see is from everyone, when it’s only from a smaller group of people that can make one choice seem to have more than the other. I think that if you don’t have enough people in a poll or survey, the statistics won’t be as accurate as they could be if you were to use more people. It’s like taking a survey to see if people prefer chocolate ice cream or vanilla ice cream. You could ask two people, if they both answer vanilla and your publish your statistics saying that 100% of people like vanilla ice cream better than chocolate, it’s inaccurate.
————————————————————————————————————————–

Activity

I would be interesting in seeing the results of how many people like/dislike/have no opinion of an anime and manga called Sword Art Online (Manga: Sword Art Online: Aincrad). I have heard lots of people have different opinions on it, so it’ll be cool to see what everyone thinks and how it averages out. I would want to survey the people who has already seen the anime or read the manga. I think that if I were to survey the people on the story line, there may be more of a positive outcome. But if I were to survey people on their opinions on the characters, the results would probably be more negative in the end. People may be biased to their friends/friend groups that they’re in. If someone who is the ‘head of the group’ claims that they didn’t like it, there is a big chance that everyone else in that group will think the same thing. I think that if I were to ask everyone at different times and locations where they are not with their main friends/ friend groups, they will probably say their actual opinions.

Protected: Tutoring #8

Protected: Tutoring #7

Protected: Tutoring #6

Protected: Tutoring #5

Protected: Tutoring #4

Protected: Tutoring #3

Everything I know about exponents

Represent Repeated Multiplication with Exponents

Representing repeated multiplication with exponents isn’t that hard. All you need to do is count how many of the numbers are and then put the number as a power. Here is an example- 5•5•5•5•5= 5⁵
So the question was multiplying five, five times in a row, but then you could simplify it to 5⁵.

How Powers Represent Repeated Multiplication

Exponents (powers) represent how many times a number is multiplying itself. So instead of writing on forever like 2•2•2•2•2•2•2•2•2•2•2•2•2•2•2•2•2•2•2•2, you could simplify that to 2²⁰. Using powers is a more simple way of writing out long multiplications. At the end, 2•2•2= 2³, meaning that if 2•2•2= 8 that should mean that 2³= 8 to because at the end they are the same question.

2³ and 3² with Models

math-everything-i-know-about-exponents-aileen-klassen
⬆Click Link for Video!⬆

The difference between 2³ and 3²

If you look at 2³ and 3² you might think that they would equal the same thing because in multiplication, when you flip the numbers around, it should equal the same thing. A common mistake is to do: 2•3=6 and 3•2=6. But it’s actually supposed to be 2•2•2=8 and 3•3=9. As you can see, the numbers are different. You can’t use short cuts like 3•2 because that isn’t the right answer. The exponent is supposed to represent how many times the base will multiply by itself. So 2³ would be 2(Base) multiplied by itself 3(Exponent) times, and that is how you would get 2•2•2=8.

Evaluating Powers with Integral Bases and Whole Number Exponents

Having integral bases doesn’t change the way you do the math. The exponent still means how many times that you multiply the number. The only difference if that if the exponent is an even number, the answer will be positive. If the exponent is an odd number, it will be negative. Here is an example: ${-5}^2$ = 25 and ${-5}^3$ = -125. Because since you know that a negative multiplied by a negative equals positive and a positive multiplied by a negative equals negative, it makes sense that a negative base to the power of a positive number will equal positive. Repeated multiplication example: (-5)•(-5)•(-5)•(-5)= 625⬅ Positive because it was multiplied and even amount of times. (-5)•(-5)•(-5)= -125⬅Negative because it was multiplied an odd number of times. It become negative because (-5)•(-5) equals $positive$ , then multiplying it by a negative again will make the answer $negative$ because you multiplied the positive answer, (-5)•(-5) and then added a third negative number (-5)•(-5)•(-5) making the answer negative. Here is a step by step: (-5)•(-5)•(-5)= 25•(-5)= ${-125}$ .

The Role of Parentheses (Brackets) in Powers

If you were to be given the questions (-2)⁴, (-2⁴) and -2⁴ you would get different answers. Even though you would get the same answer with -2⁴ and (-2⁴), writing them out in a repeated multiplication would look different. For (-2)⁴, writing it out as a repeating multiplication would look like this: (-2)•(-2)•(-2)•(-2)= 16 and writing out (-2⁴) would look like this: (-1•2•2•2•2)= -16. You get the same answers but the writing them out part is different. Because in (-2⁴), the exponent is in the brackets meaning that everything happens inside the brackets. But for (-2)⁴ it is whatever is in the brackets four times. Now for -2⁴. When you are doing this, the $-$ is a coefficient. So it represents $-1$ . You would use BEDMAS for this one, so you would do 2⁴ which equals 16, then you would do (-1)•16= -16. So the answer would be -16, which is very different from 16, so don’t mix it up!

Exponent Laws for Multiplying and Dividing Powers with the same Base

When you get a question like 2³÷2², you don’t need to use BEDMAS to find the answer. There is a simpler way you can do that. Since it is division, you can subtract the exponents so you would have $2^{3-2}$ = 2¹= 2. For multiplication, you would add the exponents instead of subtracting them: 2³•2²= $2^{3+2}$ = 2⁵= 32. So if the bases are the same you can simplify it by; Multiplication~Adding the exponents or Division~Subtracting the exponents.

Exponent Law for Raising a Product and Quotient to an Exponent

Like in the last question, for multiplication (Product Law) you would add your exponents to get a single power if the bases are the same, and for division (Quotient Law) you would subtract your exponents to get a single power if your bases are the same.

The Law for Powers with the Exponent Zero

If you think back to multiplication, you would think that 5⁰ would equal 0. But that is wrong, 5⁰=1. Here is why; with powers there is a pattern, each time the exponent goes up by one, the answer multiplies by the base, and when the exponent goes down by one, the answer divides by the base. Here is an example: 5⁵= 3125➡ 5⁴=625➡ 5³=125➡ 5²=25➡ 5¹=5 and if the pattern continues to 5⁰, that should mean that 5⁰=1 because 5÷5=1. Also if you use the quotient rule for 5²÷5² and use BEDMAS, it would be: 25÷25=1 so that would mean that $5^{2-2}$ = 5⁰=1 because it is the answer to the same question. That justifies that x⁰ will always equal 1.

Using Patterns to Prove that bases with the Exponent 0 Equals 1

Like I had explained before, the pattern is that each time the exponent rises by 1, your answer will by x times bigger than the the one before it (x as a base). So that should mean that it works the same going down, then you divide the answer by x each time the exponent goes down by one, meaning that x⁰=1.

Law for Powers with Negative Exponents

With negative exponents, you need to be able to flip the number so it’s in a fraction. Let’s use $2^{-5}$ . So right now we can put it in the fraction form: $\frac{2^{-5}}{1}$ . Now we flip it to $\frac{1}{2^5}$ . Now since it’s positive, we can use BEDMAS to solve it. So 2⁵= 32. So now we have $\frac{1}{32}$ . You can leave it like that or you can solve it. So 1÷32= 0.03125. So whenever you need have a negative exponent, you need to turn it into fraction form if it is not already and then you switch the numbers with the exponents that are negative from the top to the bottom and vice versa.

Patterns for the Negative Exponent Law

The pattern for negative exponents are the same pattern as positive exponents, the only difference is that it’s a fraction with 1 as a numerator. So here is an example of the pattern: 2³=8➡ 2²=4➡ 2¹=2➡ 2⁰= 1➡ $2^{-1}$ = $\frac{1}{2}$ ➡ $2^{-2}$ = $\frac{1}{4}$ ➡ $2^{-3}$ = $\frac{1}{8}$ . So the pattern continues, but instead as a fraction instead of a whole number.

Applying Exponent Laws to Integral and Variable Bases

For Variable Bases-
x⁸=x⁸ You can’t change it because you don’t know what x equals

y⁰= 1 because you know no matter what number, that ANYTHING to the power of 0 will equal one.

For Integral Bases-

They work the same as positive bases, just that you have to beware that if it’s to an uneven power, it will be negative, it it is even the answer will be positive.

Identifying an Error in a Simplification of an Expression Involving Powers

If you see something like 3(2³) some people might doing something like = 6³. THAT IS WRONG.
You need to stick to the rules of BEDMAS and do the exponents first, so you should do- 2³=8= 3•8=24. So 3(2³)= 24.

Using the Order of Operations on Expressions with Powers

For example you could use 5(2³). So because of BEDMAS, you have to do do the exponent first by doing 2³ which equals 8. Then you will end up with 5(8) and now you just need to multiply 5•8=40. So the answer to 5(2³)= 40.

Determine the Sum and the Difference of Two Powers

All you need to do is BEDMAS to calculate the Sum and the Difference.
Ex: 5²+2³= 25+8= 33
5²-2³= 25-8=17

Identifying an Error in Applying the Order of Operations in an Incorrect Solution

This is wrong:
5²+5=
10²= 100

So you would have to do the exponents first and then you could do the addition second.

Correct way:
5²+5=
25+5= 30

So you can see if you do it the wrong way, you can get completely different numbers. In the examples you could have gotten 30 or 100.

Using Powers to Solve Measurement Problems

For example, if you need to find the grey part of the square. You can see that there is a white square $math-square$ inside the grey square, meaning that you want to take out the area of the white square. So first, you will need to calculate the area of the the grey part: 5cm²= 25cm². Now you can calculate the area of the white square: 1cm²= 1cm². So now you can subtract the area of the white square from the area of the grey square: 25cm²-1cm²= 24cm². So that means that the area of the grey space is 24cm²

Using Powers to Solve Growth Problems

Using exponents can be helpful for growth problems. For example, two bacteria are in a jar, each hour they double. So you could use 2² for the first hour, then it would be 4², 8², 16²… So it can show how it duplicates every hour and you would be able to figure out how many bacteria are in the jar. It makes figuring out the answer a lot easier and faster. It also helps you understand the question more when you think of it with powers.