Math 10

Modeling Polynomials

minjih2015

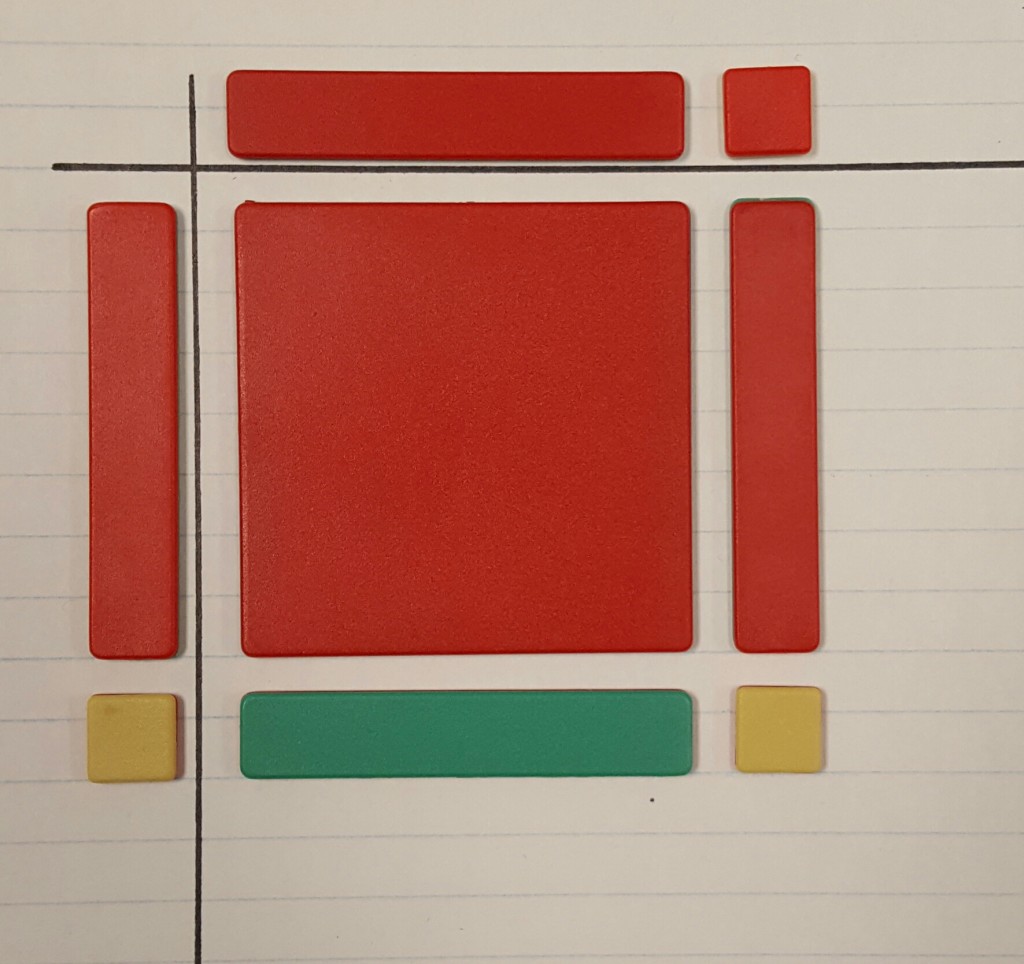
Before using the algebra tiles to model the mathematical expressions, I chose each colours of the tiles to represent what it meant.

Key :

2016-11-07-14-43-57

  • (x+1)^2

2016-11-07-14-54-54

(x+1)^2

= (x+1)(x+1)

= x^2+x+x+1

= x^2+2x+1

I expanded the expression (x+1)^2 in to (x+1)(x+1), because this makes it easier to understand why the algebra tiles are labled like that. There are one red stick and one red small square on each of the two sides. In the middle, there are red large square, two red stick, and 1 red small square.

  • (x-1)^2

2016-11-07-14-55-36

(x-1)^2

= (x-1)(x-1)

= x^2-x-x+1

= x^2-2x+1

This equation is similar to the prior. The only difference is that this equation has a -1.

To solve this equation, I expaned (x-1)^2 in to (x-1)(x-1).

  • (x+1)(x-1)

2016-11-07-14-56-08

(x+1)(x-1)

= x^2-x+x-1

= x^2-1

I put a red stick and a red small square on the top side, and a red stick and a yellow small square on the left side. Then, I could know which alegebra tiles had to go in to the middle. A red big square, two sticks that are different colours, and one yellow small square.

  • (x-1)^3

2016-11-07-14-55-36

(x-1)^3

= (x-1)(x-1)(x-1)

= (x^2-x-x+1)(x-1)

= (x^2-2x+1)(x-1)

= x^3-2x^2+x-x^2+2x-1

= x^3-3x^2+3x-1

I couldn’t model this expression because it was cubed, so I had to solve it by doing two steps. First, I wrote it down like this, (x-1)(x-1)(x-1). I multiplyied the two binomials together, and that gave me (x^2-2x+1). Then, I multiplied the trinomial and (x-1). Finally, I simplified the expression, and the simplified expression was x^3-3x^2+3x-1.

Trigonometry

minjih2015

In math class, we are learning about trigonometry. From yesterday’s lesson, we learned that it is about a relationships involving lengths and angles of triangles.

 

2016-10-25-13-58-01-280788450

The longest side, AB, is called the hypotenuse (hyp).

The side opposite the angle of A, AC, is called the opposite (opp).

The third side of the triangle, BC, is called the adjacent (adj).

Sin A = opposite / hypotenuse

Cos  A= adjacent / hypotenuse

Tan  A = opposite /  adjacent

 

When using calculators, I could know the ratio by pressing the buttons ‘sin’, ‘cos’, or  ‘tan’ .

To know the angle, I would press the buttons ‘sin-1’, ‘cos-1′, or tan-1’.

 

Measurement

minjih2015

Today in math class, we started learning about measurements. We went outside and found objects that can be used to measure the length and volume. My partner and I found 2 branches, a cup, and some little plastic sticks. We then, tried to measure our height by using the objects that we found. My height was almost 5 times the branch. When we finished talking about measurements, we went back to our class and watched two videos, one was about the history of measurement, and the other one was about history of numbers. Today’s class was really fun and it was a great way of learning about measurements. I could understand very easily.

Exponential Graph

minjih2015

Today in math, each group were assigned to graph an exponential equation.

Our group had to graph the equation  y.

We first made a table of values with x values from -10 to 10, then found the y values and plotted the points. We connected the points and the graph looked like this.

20160927_150950

It had a curved line shaped as ‘L’ and most of the y values were closed to zero. The distance between the points was far when the x was negative, and the distance was short when the x was positive. This is because, when x is a negative number, y decreases rapidy. However, when x is a positive number, y decreases slowly. Even though it gets smaller and smaller, y never becomes zero.

After our group finished, we compared each other graphs with group 8. This is what their graph looked liked. %ec%ba%a1%ec%b2%98

Their equation was y.  The equation was the same except they had an negative exponent. Therefore, their graph is a reflection on the y-axis. If our graph looked like an ‘L’, group 8’s graph was the appearence of the opposite of ‘L’.

From this activity, I could easily understand integral exponents by looking at an visual graph of how the numbers are related. For example, (1/2)^3 is 1/8 while (1/2)^-3 is 8.