Tell
The thing that I learn this week are arithmetic sequence, arithmetic series, geometric sequences and geometric series.
In an arithmetic sequence, the difference between consecutive terms is constant. This constant value is called the common difference.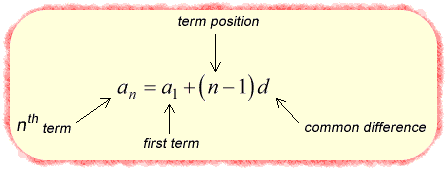
The General Term of an Arithmetic Sequence is :tn = t1 + d( n – 1 ) When the terms of an arithmetic sequences are added together it is known as an arithmetic series.
The sum of n terms of an arithmetic series: Sn = n(t1+tn)/2 or Sn = n[2t1+d(n-1)]/
A Geometric sequence is formed by multiplying each term after the 1st term by a constant, to determine the next term. The constant is the common ratio,r, of any term by the preceding term. tn=a*r^(n-1) A geometric series is the sum of the terms of a geometric sequence. So the sum of n terms of a geometric series. Sn=t1(1-r^n)/1-r , r not = 1
