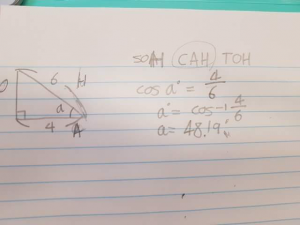Month: November 2017
Week 12 in Math 10
It is the review of Trigonometry unit in math 10. I reviewed the rules about sine, cosine, and tangent. (I drew every picture and made it)
Week 11 in Math 10
What we learned Week 11 in Math 10 is to factor when the degree of a polynomial is more than 2. (for examples 4, 6, et cetera.) The bases of factoring polynomials are C, D, P, E, and U.
C has used the polynomial which can be divided into common factors.
3x2 + 6x = 3x(x + 2)
D is differences of squares, it is used when the polynomials are perfect squares.
16 – x2y2 = (4 – xy)(4 + xy)
P is to find the pattern of the polynomial.
x2 + 6x – 7 = (x + 7)(x – 1)
E is used by easy polynomials which can be used the easiest pattern.
x2 + 4x + 4 = (x + 2)2
U is used by ugly polynomials. (We usually use a square to factor.)
6x2 + 13x + 6 = (2x + 3)(3x + 2)
We can use the bases and solve the polynomials which degree is bigger than 2. We also learned the pattern about a degree. When the degree is bigger than 2, other exponents of x must be half of the degree if there are not any common factors, and it cannot be factored if it is not.
For an example, 32x4 – 2 can be divided by 2 (Greatest common factor) and it becomes 2(16x4 – 1). 16x4 and -1 are perfect squares, we can use D. 2(16x4 – 1) = 2(42 + 1)(42 – 1). 42 – 1 is even perfect square which can be used D, but 42 + 1 is not because the binomial must have ONE minus sign. 2(42 + 1)(42 – 1) = 2(42 + 1)(2x + 1)(2x – 1). So, the answer is 2(42 + 1)(2x + 1)(2x – 1).
Week 10 in Math 10
What I learned this week in Math 10 is to factor trinomials easily. It seems more difficult, but trinomials that can be factored if we find and use the pattern, it is similar to simplify polynomials. I could find the pattern of factoring other trinomials. It is not different to find and divide trinomials by the greatest common factor (GCF) on trinomials from simplifying polynomials.
Factoring with Common Factor: find greatest common factor and divide the trinomial by GCF.
Example: 3x2 + 6x + 9 = 3(x2 + 2x + 3)
Factoring the square binomials: the terms in binomials are perfect squares.
Example: 9x2 – 4 = (3x + 2)(3x – 2)
BUT, it is impossible => 9x2 + 4
Factoring simple trinomials: find the factors of last term and add them, and find a pair which is become a number of middle term (coefficient of x)
Example: x2 + 4x +3 = (x+3)(x+1)
Factoring UGLY trinomials:
The most important term in the trinomial in the picture is the first term that is the coefficient of x2 and the term that is just a number. After he coefficient of x2 and the last numbers are multiplied, we can find the factors of the multiplied number. (1, 24), (2, 12), (3, 8), and (4, 6). The numbers are added by their pair (1+24 = 25), (2+12 = 14), (3+8 = 11), and (4+6 = 10) and we can find the middle term (the coefficient of x, 11) 3+8 = 11. Factors of 6 are (1, 6) and (2, 3). Factors of 4 are (1, 4) and (2, 2). 1×3 (= 3) + 4×2 (= 8) = 11.
So, 6x2 + 11x + 4 = (3x + 4)(2x+1)
Complex and Compound-Complex Sentences
She was a famous writer of popular novels and she was preparing to write a new novel.
Although she was an author of famous novels, it was not easy to create stories and write her imagination on papers until the sky turned dark.
Unless an interesting or special situation happened to her, it seemed impossible to write her own story even if she focused on only making a story for a few days.
When she did not have any ideas before, she walked around her town and talked neighborhoods.
Meeting people was interesting to her because she thought their talk might help or be an idea of her story.
When she prepared to go outside, she realized the outside turned dark a long time ago and there was nothing including a small movement.
She tried to go outside whether there was something or not, but she felt the just cold atmosphere and went back to her study again.
Although a few pieces of fire woods on the stove were still burning, she felt colder than outside.
She gave up writing new stories and just started to read her favorite novels in the study because her will was still staying in cold outside and she should try to forget the cold.
Another word of imagination was isolation because she thought reading a book is same to make another world in a human head.
While she concentrated on books, it was being easier to forget her anxiety including a problem of her people.
She decided to become a writer by attraction to books, and she hoped to make other medicine of forgotten stress while she read books.
Week 9 in Math 10
We started to learn Polynomial Factoring Unit in this week. When we solve the questions, it takes a lot of time for simplified, so we tried to find the pattern or the method of simplifying polynomials by ourselves. The picture is one of the method to solve binomials easily. x2 is a square, x is a stick, and 1 is a smaller square.
2x and +1 can be each multiplied by x-3.
2x * (x-3) = 2x * x – 2x * 3 = 2x2 – 6x, 1 * (x – 3) = x -3. So, 2x2 – 5x – 3.
We solve the product of polynomials, I found the pattern how to simplify the polynomials easily.
- When x is multiplied by x, just multiply the coefficients firstly and multiply x2 too.
- (ax + b)(cx + d) => (bc + ad)x
- Just Multiply another number in the polynomial as step 1.
week 8 in math 10
What we learned in this week is to solve problems using SOH, CAH, and TOA. For example, on the problem, we already know what is the adjacent and the hypotenuse, so we can use cosine. We need to find what is a, so the way is
cos a = adjacent/hypotenuse
a = cos-1 adjacent/hypotenuse
The adjacent is 4 and the hypotenuse is 6.
a = cos-1 4/6
so, a is similar to 48.19