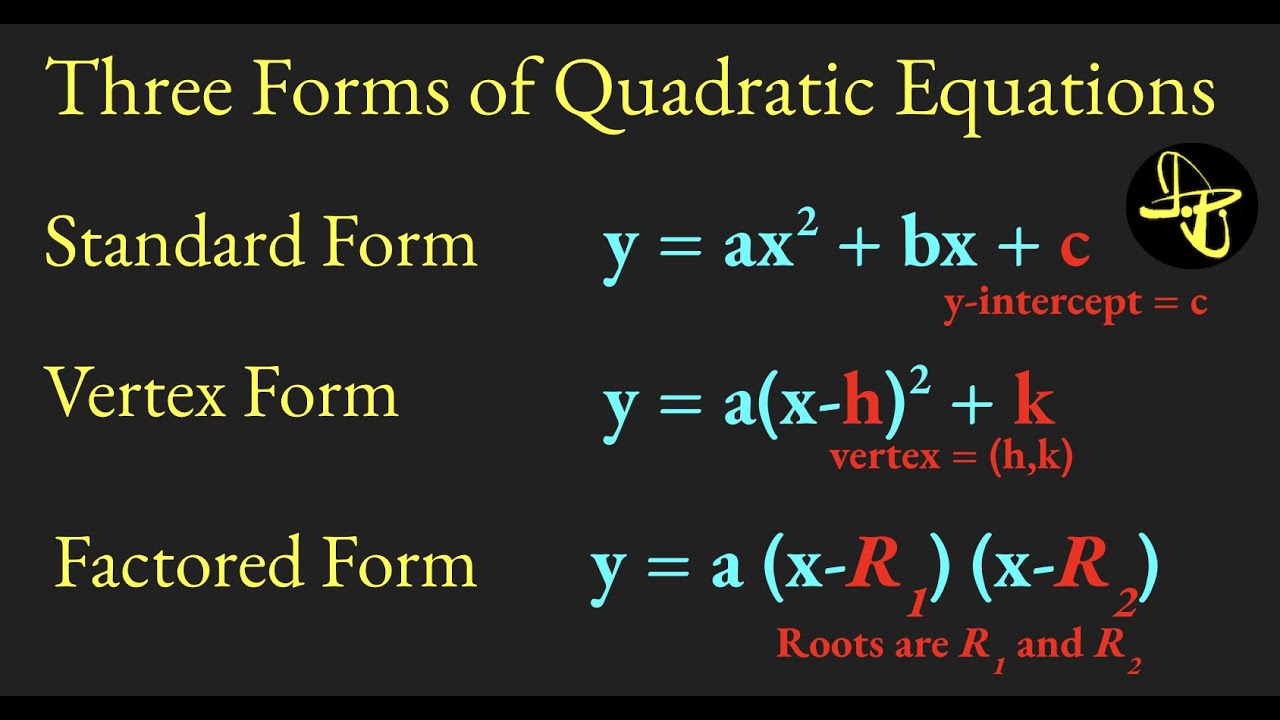
I choose what we learned about going from general to standard form quadratic functions and how the different forms can be used to graph the function. I choose this topic because it allows you to graph and figure out the different parts of a quadratic function with different form of the function instead always converting it to vertex form and we also learned about converting general to standard form which is nessacary when using standard form quadratic functions. We learned that the factored form helps in quickly identifying the roots (zeros) of the quadratic equation, which are where the function intersects the x-axis. It’s useful for solving quadratic equations and getting the vertex by adding the two roots and diving by two to find the x cordinate of vertex which can be put in the equation to find the y-cordinate. the general form provides a comprehensive view of the quadratic equation, showing the coefficients of x², x, and the constant. It’s often used in algebraic manipulations, such as completing the square to find the vertex form or solving systems of quadratic equations. the Standard form highlights the vertex of the quadratic function, which is the highest or lowest point on its graph depending on the quality of the coefficient. It’s crucial for graphing quadratic functions accurately and understanding transformations like shifts, stretches, and compressions. This is also how you convert standard for to general form

Converting from Standard Form to General Form (With Coefficient on )
Starting with the standard form :

1. Start with the Standard Form:
2. Expand the Equation:
3. Group the Terms: Group the quadratic term and the linear term together:
4. Factor Out the Common Factor
5. Complete the Square: Add and subtract inside the parentheses:
6. Factor and Simplify:
7. Distribute \a  and Simplify Further:
8. Combine the Constants:
9. General Form:

Converting from Standard Form to General Form (Without Coefficient on \( x^2 \))
Starting with the standard form

1. Start with the Standard Form:
2. Complete the Square: Add and subtract inside the parentheses:
3. Factor and Simplify:
4. General Form:

In both cases, you end up with the quadratic function in general form, which is , where and when there is a coefficient on the term, and and when there is no coefficient on the term.

Let’s work through an example of converting a quadratic function from standard form to general form step by step.

Example:
Convert the quadratic function from standard form to general form.

Steps to Convert from Standard Form to General Form:
1. Start with the Standard Form:
2. Factor Out the Coefficient : Since , we factor out
3. **Complete the Square Inside the Parentheses:**
– Add and subtract inside the parentheses:
– Rewrite the equation:
4. Distribute 2 and Simplify: 
5. **Combine Constants:**

Now, the quadratic function is in general form: . In this form, and , which represent the vertex of the parabola.

So, the quadratic function in standard form is equivalent to in general form.

link