Week 18 – Top 5 Things I Learned
Over this semester of Pre-Calculus 11, we covered many units. But the most important thing I learned, was how to work smarter and not harder. There’s always an easier way to do every problem, and a catchy little acronym to go with it. Each unit we covered brought new insight and challenges into my world of math and problems that stretched my brain as far as possible. But I didn’t hate it. Can Divers Pee Easily Underwater? I know the answer to that question. Does Ms. Burton love Slurpee’s as much as we do? Absolutely. Pre-Calculus 11 had it’s many ups and downs, but i learned so many new things that I’ll take with me into the future. The top 5 lessons I learned this year were:
Arithmetic Sequences:
Arithmetic sequences are a group of numbers (i.e. 4, 9, 14, 19, 24…) that increase by a steady amount. In this case 5. Using the equation we can find any number within the sequence, with
representing the unknown number,
represents the first number in the sequence,
represents the difference between numbers, and
being the number we’re trying to find. The plugged in equation would look like
which in turn is
.
With this equation you can implement any number of the sequence into the equation to find it’s value.
Graphing Quadratic Equations:
If we factor a quadratic equation, we can find the roots, as well as the axis of symmetry. With this information, we can draw a quick graph depicting what our parabola would look like.
ex. Graph the equation
graphing the equation with only the factored form won’t give us a complete parabola, but it will give us enough information to get a rough idea.
$latex y=-2(x+5)(x-2)
replace the “y” with 0.
0=-2(x+5)(x-2)
rearranging the bracketed “x’s” will give us our roots
(x+5) -> x=-5, (x-2) -> x=2
with the x-intercepts, we know that when graphed will be in the format (x,y). But since the x-intercept is where the parabola crosses the x-axis, the y-intercept will equal 0. The x-intercepts will be (-5,0) and (2,0). The axis of symmetry is half way between both roots, so the AOS will be x=-1.5.
Using the Discriminant:
The discriminant is the area under the square root in the quadratic equation:
if you have a quadratic equation (equation equal to zero with 3 distinct parts), you can use the quadratic formula to solve. Depending on the answer, we can figure out whether the equation will have 1,2 or 0 solutions. With a quadratic equation in the format we can substitute the a, b and c values into the discriminant equation to find out how many roots.
Parabolas:
Graphs of quadratic functions all have the same shape which we call parabola, that can be graphed to give us information such as the roots, y-intercept, axis of symmetry, etc. In order to find this, we have to first graph it using some of these easy tricks.
A translation is an image of the original parabola, but moved either horizontally or vertically from the original parent equation
Depending on the equation, you can determine where the parabola has moved on the number line.
If the coefficient of changes, the parabola will either stretch or compress. A coefficient less than 1 will cause the parabola to compress, while a coefficient more than 1 will cause it to stretch.
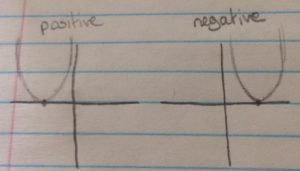
If the coefficient is negative the parabola will open down, while if it’s positive it will open up.
In the case that your equation looks similar to , with your numbers in brackets, then the “c” will effect whether your parabola translates left or right. If the number is positive, it will translate left, and if it’s negative it will translate right. It does not follow the general idea that negatives go left and positives go right.
Sine and Cosine Law:
Sine and Cosine Law are used to solve for angles and sides of a triangle that isn’t a right triangle.
Sine Law is used when you’re able to use the formula . In order to use the formula you must have all values of one fraction, and half of 1 other. This way, you can solve for an angle/side you’re missing. It can be any part of the formula, as long as one + half of another is given.
Cosine law is used when two sides and one angle are given to you, but you’re trying to find the missing side. The formula used is for finding a side, or
.