Archive of ‘Grade 11’ category

Enough
by Sarah Lilley
Why are girls beautiful until they’re stronger than you?
Ashamed to be around her
Once she’s able to do it better than you do
You refuse to be called anything less than sir
Since her IQ is higher than the mountain
That you’re forcing her to climb
In order to be an overflowing fountain
That you make her struggle for every dime
It’s nothing short of cruelty
Your sympathy is less than treacle
It fails to see her beauty
Because she is less than equal
And the path ahead is rough
But she is enough
This poem is by author Sarah Lilley, addressing the growing case against the enforcement of gender roles. The author sends a clear message that she does not agree with the stereotypes that are placed upon women to submit to men as the more dominant gender.
The poem is written in the form of a sonnet (abab cdcd efef gg) to create a rhyme scheme that fits her poem. The poem’s tone is very judging, almost challenging the reader to contradict the idea. To prove that what she’s stating is wrong. In line 10 it states “your sympathy is less than treacle” meaning that unless you, the reader, make a stand for equality among genders your sympathy will not make a difference.
A metaphor is used in lines 5-6 comparing the woman’s IQ to a mountain that she’s being forced to climb. This means that society has placed many obstacles in front of her goals that she must overcome before moving forward, like a mountain for instance. She must “climb” a difficult mountain of social hierarchy before she’s able to reach her goals as a woman. No matter how smart she may be, it does not matter to people who believe there’s a man just as capable.
The theme of the poem is very feminist. It addresses the underlying problems that our society has placed on the backs of women to carry. It’s made it that much more difficult to get a woman to the same level as a man in the work force because “in order to be an overflowing fountain you make her struggle for every dime”(line 8).
Women are not able to reach their full potential unless they are treated with the same respect as men.
Over this semester of Pre-Calculus 11, we covered many units. But the most important thing I learned, was how to work smarter and not harder. There’s always an easier way to do every problem, and a catchy little acronym to go with it. Each unit we covered brought new insight and challenges into my world of math and problems that stretched my brain as far as possible. But I didn’t hate it. Can Divers Pee Easily Underwater? I know the answer to that question. Does Ms. Burton love Slurpee’s as much as we do? Absolutely. Pre-Calculus 11 had it’s many ups and downs, but i learned so many new things that I’ll take with me into the future. The top 5 lessons I learned this year were:
Arithmetic Sequences:
Arithmetic sequences are a group of numbers (i.e. 4, 9, 14, 19, 24…) that increase by a steady amount. In this case 5. Using the equation  we can find any number within the sequence, with
we can find any number within the sequence, with 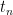 representing the unknown number,
representing the unknown number,  represents the first number in the sequence,
represents the first number in the sequence,  represents the difference between numbers, and
represents the difference between numbers, and  being the number we’re trying to find. The plugged in equation would look like
being the number we’re trying to find. The plugged in equation would look like 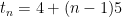 which in turn is
which in turn is  .
.
With this equation you can implement any number of the sequence into the equation to find it’s value.
Graphing Quadratic Equations:
If we factor a quadratic equation, we can find the roots, as well as the axis of symmetry. With this information, we can draw a quick graph depicting what our parabola would look like.
ex. Graph the equation 
graphing the equation with only the factored form won’t give us a complete parabola, but it will give us enough information to get a rough idea.
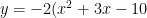
$latex y=-2(x+5)(x-2)
replace the “y” with 0.
0=-2(x+5)(x-2)
rearranging the bracketed “x’s” will give us our roots
(x+5) -> x=-5, (x-2) -> x=2
with the x-intercepts, we know that when graphed will be in the format (x,y). But since the x-intercept is where the parabola crosses the x-axis, the y-intercept will equal 0. The x-intercepts will be (-5,0) and (2,0). The axis of symmetry is half way between both roots, so the AOS will be x=-1.5.
Using the Discriminant:
The discriminant is the area under the square root in the quadratic equation:

if you have a quadratic equation (equation equal to zero with 3 distinct parts), you can use the quadratic formula to solve. Depending on the answer, we can figure out whether the equation will have 1,2 or 0 solutions. With a quadratic equation in the format 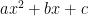 we can substitute the a, b and c values into the discriminant equation to find out how many roots.
we can substitute the a, b and c values into the discriminant equation to find out how many roots.
Parabolas:
Graphs of quadratic functions all have the same shape which we call parabola, that can be graphed to give us information such as the roots, y-intercept, axis of symmetry, etc. In order to find this, we have to first graph it using some of these easy tricks.
A translation is an image of the original parabola, but moved either horizontally or vertically from the original parent equation 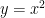

Depending on the equation, you can determine where the parabola has moved on the number line.
If the coefficient of 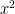 changes, the parabola will either stretch or compress. A coefficient less than 1 will cause the parabola to compress, while a coefficient more than 1 will cause it to stretch.
changes, the parabola will either stretch or compress. A coefficient less than 1 will cause the parabola to compress, while a coefficient more than 1 will cause it to stretch.

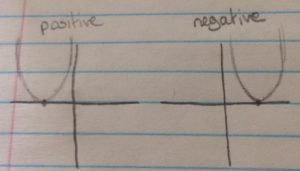
If the coefficient 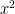 is negative the parabola will open down, while if it’s positive it will open up.
is negative the parabola will open down, while if it’s positive it will open up.

In the case that your equation looks similar to  , with your numbers in brackets, then the “c” will effect whether your parabola translates left or right. If the number is positive, it will translate left, and if it’s negative it will translate right. It does not follow the general idea that negatives go left and positives go right.
, with your numbers in brackets, then the “c” will effect whether your parabola translates left or right. If the number is positive, it will translate left, and if it’s negative it will translate right. It does not follow the general idea that negatives go left and positives go right.
Sine and Cosine Law:
Sine and Cosine Law are used to solve for angles and sides of a triangle that isn’t a right triangle.
Sine Law is used when you’re able to use the formula  . In order to use the formula you must have all values of one fraction, and half of 1 other. This way, you can solve for an angle/side you’re missing. It can be any part of the formula, as long as one + half of another is given.
. In order to use the formula you must have all values of one fraction, and half of 1 other. This way, you can solve for an angle/side you’re missing. It can be any part of the formula, as long as one + half of another is given.

Cosine law is used when two sides and one angle are given to you, but you’re trying to find the missing side. The formula used is  for finding a side, or
for finding a side, or  .
.

This week we learned how to use Sine Law and Cosine Law to find the angles and sides of triangles that aren’t right angles.
Sine and Cosine Law are used to solve for angles and sides of a triangle that isn’t a right triangle.
Sine Law is used when you’re able to use the formula  . In order to use the formula you must have all values of one fraction, and half of 1 other. This way, you can solve for an angle/side you’re missing. It can be any part of the formula, as long as one + half of another is given.
. In order to use the formula you must have all values of one fraction, and half of 1 other. This way, you can solve for an angle/side you’re missing. It can be any part of the formula, as long as one + half of another is given.

Cosine law is used when two sides and one angle are given to you, but you’re trying to find the missing side. The formula used is  for finding a side, or
for finding a side, or  .
.

This week we spent some time reviewing trigonometry from grade 10, so that we would be refreshed for our trigonometry unit this year.
Important things to remember:
3 main ratios in trig are Sine (sin), Cosine (cos), and Tangent (tan)
An abbreviation we use to remember this is SohCahToa
SohCahToa is used to find angles of right triangles.
For example: H=8, O=4. Find angle x.

This diagram labels the hypotenuse, opposite and adjacent, but not all diagrams are labeled. Too figure out which is which is easy!
Hypotenuse will always be your longest side, and is usually straight across from your right angle.
Adjacent is the side directly connecting your right angle and missing angle.
Opposite is the side leftover or directly across from the missing angle.
In our abbreviation Soh Cah Toa: “S” stands for Sine, “C” stands for Cosine and “T” stands for Tangent. Other than these, your smaller letters all stand for something: Your “o”pposite, “h”ypotenuse and “a”djacent.
Knowing where each side is can help us dictate whether to use Sine, Cosine or Tangent to solve for the angle.
In our diagram, we’re given the length of the hypotenuse and the opposite. Knowing that we decide to use “Soh” from our abbreviation because it’s the only one with opposite and hypotenuse.
To find the angle we use the “sin” button on our calculator, but the inverse function of it because we’re solving for the angle and not a side.
When punched in the equation should look like 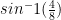
If you were instead solving for a side, the regular “sin” button would be used instead.
This week we learned how to add and subtract rational expressions. These are a bit harder than multiplying and dividing because the denominator has to be the same throughout the expression.
Steps to simplify:
- determine lowest common denominator (LCD)
- rewrite each fraction as an equivalent fraction with LCD
- Combine numerators by adding/subtracting
- reduce if possible
For example:  where “x” cannot equal 0 or 4.
where “x” cannot equal 0 or 4.
- For this expression, the least common denominator would be
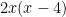 because neither denominator can be simplified any farther or multipled to create a common denominator, so we multiple the two denominators together to create one LCD.
because neither denominator can be simplified any farther or multipled to create a common denominator, so we multiple the two denominators together to create one LCD.
- Since we’re multiplying the bottom, we also have to do the same to the top, so the equation becomes:
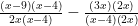 .
.
- This simplifies to

- The final simplified expression will be
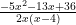

In 1953, a book was written that challenged certain conditions in the United States so extremely, that it is still relevant to this day. Fahrenheit 451 by Ray Bradbury is a book that takes place in the dystopian future where Guy Montag lives as a fireman burning the most illegal of commodities, the printed book. The firemen were created to destroy books and “be custodians of our peace of mind” so that people would not dread being inferior; “official censors.” Guy lives in a world where people see the world through mindless chatter from television instead of the ideas that books present. Guy meets Clarisse, a girl who changes his perception of what his world is supposed to look like and leaves him to question everything he’s ever known. Wall-E is an animated movie that follows a robot name Wall-E, who works as a clean up crew on Earth after humanity is forced to leave due to pollution and the buildup of garbage. The little robot’s world is turned upside down when another robot name EVE arrives from the Axiom, searching for any signs of life on Earth. Time to do Everything but Think by David Brooks is an article about the excessive distractions surrounding people and how it prevents us from “diving deep.” Censorship was used as a suppressant for anti-intellectualism in Fahrenheit 451 and is still relevant to today’s society. In Fahrenheit 451 censorship is explored through mass media, propaganda, and the censorship of war.
Fahrenheit 451 has a focus on the importance of television to the people. Because of this, the citizens are easily controlled by what they watch on tv. Censorship is used to keep people from expressing their own opinion on a matter. If you get rid of the thing, in this case books, that causes turmoil among the people, it makes them much easier to control. But as soon as books are brought back into the picture, “a book is a loaded gun in the house next door.” As a person is able to express intellectualism and their thoughts on a matter, more people are able to speak up. This causes turmoil between minorities as people try to voice opinions without hurting others. Removing books made people turn to television as their next source of entertainment, where the government can easily manipulate what people are seeing. As people constantly are exposed to the same ideas repeatedly, they begin to believe what they see and hear. In Time to do Everything but Think, it states that “if you are consumed by the same information loop circulating around everyone else, you don’t have anything to stimulate you into thinking differently.” In Wall-E a large corporation known as ‘Buy n’ Large’ controls all of humanities lives. This corporation chooses what the people see, hear, and are exposed to, causing them to blindly follow exactly what it wants. This connects to real life where in the Unites States the Sinclair Broadcasting Group’s broadcasting channels reach 40% of all Americans. The Sinclair Group is conservative, Pro-Trump and consistently uses it’s platforms to expose Americans to the group’s political views. Fahrenheit 451 demonstrates how the mass media can manipulate a viewer’s perception of something. For example, Jesus is used in commercials to “mak[e] veiled references to certain products every worshipper needs” and abuse people’s beliefs for a marketing scheme. Mass media is used daily to censor topics that might offend certain groups, but also voicing their own opinions.
Propaganda is the use of media to promote a certain political stance or point of view. In Fahrenheit 451 Mildred’s friend’s believed Winston Noble was the right man to vote for only because the television viewed him as the better choice. He was portrayed as the better candidate, with good looks and a name that rolled right off the tongue. The television did not advertise the other candidate, Hubert Hoag, as anything but a fat and short man who didn’t know how to dress. The television coverage was made to downgrade Hubert and show-off Winston. Media today uses similar tactics when it comes to politics. When a person is running for office, commercials often come out mocking a certain candidate, while uplifting another. In Wall-E the Axiom is advertised as a few month space cruise, where people can get away from the polluted Earth while the Wall-E robots clean up their mess. However, ‘Buy n’ Large’, the corporation that runs it failed to acknowledge the unavoidable truth that nobody would be able to return to Earth. The corporation only advertised their products and failed to report on Earth’s impending doom. Propaganda is used however the distributor wants it to be used, always for their own personal gain.
War is a serious issue people all over the Earth face. However, the news only chooses to show what it believes is important. Censorship is used in Fahrenheit 451 to make light the subject of war. The people don’t even know what’s happening in the war, only that their men go to fight. Nobody knew the severity of the war and how close to home it was until “it began and ended in [an] instant.” The people were shielded from the horrors of war because it would cause unrest and anxiety. The country’s technological control over the people would diminish if a major war close to home was announced. Today, the news chooses to show only certain events of war in the world. It decides what it believes is important and broadcasts it. Often enough this causes one minority to be deemed ‘the enemy’ as it’s broadcasted that their home country is the reason for the strife. It fails to recognize the important topics and only show the destruction out of context. War is a very guarded topic in Fahrenheit 451 because it would cause division among minorities that will disagree on how to handle the situation, thus questioning the government’s power.
Fahrenheit 451 remains a stable in today’s literature as it addresses censorship in mass media, propaganda and censorship of war. Mass media is used to reach the masses without having to leave their house. After being exposed to this for long periods of time the people start to believe that what they’re seeing and hearing is correct. They stop ‘diving deeper’ and choose the easier option, which is that what’s shown on the television is what they should believe and disregard any other opinion. The government used propaganda to reach the people with their political views and point of view. Commercials are often used to distribute information the broadcaster sees fit and often includes paid infomercials about political candidates. People are exposed to certain view points with reasons to back up the arguments, leaving the viewer with that candidate in mind oppose to choosing for themselves. War is a topic shied away from by most people, as it is a fragile topic that leads to disagreement among people. Censorship has been utilised throughout history to limit what a viewer sees on television or in real life. It protects minorities from discrimination and shields children from adult content, but it also fails to value intellectualism. Censorship limits the intellectual freedom people have and is demonstrated as such in the novel Fahrenheit 451.
This week we learned how to multiply and divide rational expressions, which are much simpler than adding and subtracting.
It’s easiest to explain how to do the question as an example so,
e.x. 
When multiplying and dividing, you can cancel out expressions that are in both the numerator and denominator. In this case: 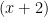 and
and 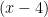
After doing this, your equation should look like 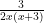
Because you can’t factor the expression anymore, that’s as far as it can be simplified.
Another thing to always do with expressions is label your restrictions. In this case, the restrictions are number that can’t be used in the place of variable “x” that would cause the denominator to become 0.
For this equation x cannot equal 4, -3, 0, -2
Link to article:https://www.newyorker.com/magazine/2018/05/21/prince-harry-meghan-markle-and-royal-romance
I chose this article because the royal wedding of Prince Harry and Meghan Markle is right around the corner, and i wanted more insight about the couple. The author writes in a very descriptive way, giving the reader facts and knowledge about past royal couples and the controversy with certain pairs based on race, religion, ethnicity, etc. I knew of Meghan Markle from the tv show she starred in, Suits, but was not made aware of her attachment to Prince Harry until the past year. The article addressed the past women of the royal family and how race, class, and marital status is becoming less important, replaced with wealth. The pairs love story will be receiving a tv movie called “Harry and Meghan”, which will follow their romantic journey over the years.
This week we learned how to graph quadratic reciprocal functions, and learned some new vocabulary.
quadratic reciprocal functions will look one of three ways when graphed:
- the parent function only touches the x-axis in one spot

- the parent function doesn’t touch the x-axis at all

- the parent function crosses the x-axis in two places

When graphing the parabola, you first find the asymptotes, invariant points, and then the location of the hyperbola.
Asymptotes are located at where the parabola crosses the x-axis. At the x-intercept(s) you can drop a dotted line vertically, and a dotted line horizontally. Depending on how many x-intercepts you have will dictate how many asymptotes you have.
Dotted in red:
Invariant points are located where your parabola meets the points on the y-axis 1 and -1. The parabola can have 0-4 invariant points. These dictate where you’ll draw the hyperbola.
Circled in blue:
Your hyperbola is the graph of the reciprocal of your original equation. For example:  becomes
becomes 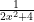
When you graph the hyperbola, there’s no need to graph each individual point. Instead, you can just draw a curved line going through your invariant point and approaching zero.
Colored in purple:
This week we learned how to solve systems algebraically with more than one variable.
For example: x+6y=4 and 0=x-4y
In these types of systems you used steps.
- Isolate a variable
- Plug the equation for that variable into the second system
- solve for that variable
- plug variable into original equation, solve for the missing variable
- Check!
This may not make complete sense in steps, so I’ll demonstrate using the first example.
- x+6y=4 can be rearranged to make x=4-6y
- next you plus your new equation x=4-6y into your second equation: 0=x-4y which turns into 0=(4-6y)-4y. This becomes y=2/5.
- Next, we plug our value for y into our original first equation x+6y=4, which becomes x+6(2/5)=4
- Simplifying the equation: x=1.6
- Check y=2/5 and x=1.6: x+6y=4 -> 1.6+6(2/5)=4 Yes! 0=x-4y -> 0=1.6-4(2/5) Yes! So we know we did it correctly













