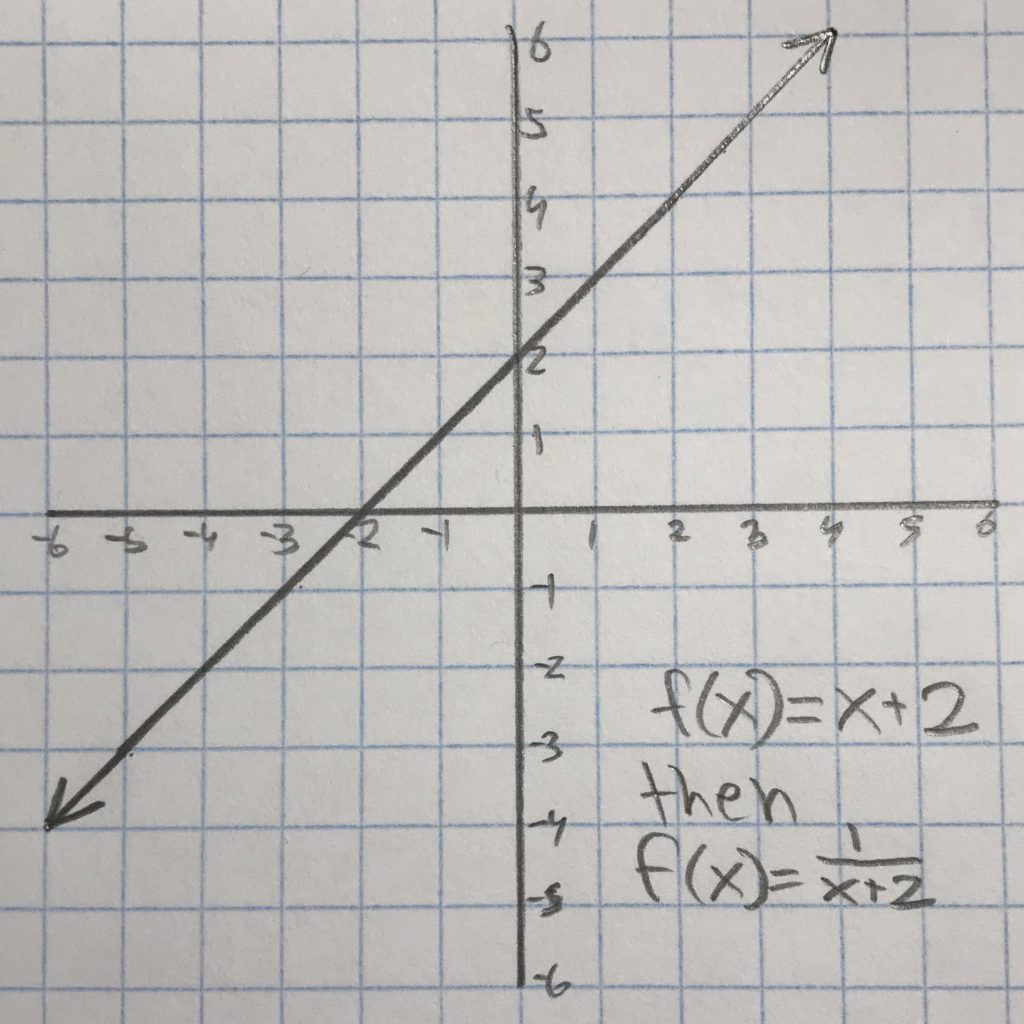LIT Circle Discussion #2
Reply
Category Archives: Uncategorized
LIT Circle Discussion #1
The Pedestrian Persuasive Video Assignment
Loading...
Reload document 
Open in new tab For the teens of generation z, the future of tomorrow looks to be engulfed by technology. From technology in the workplace to assistive technology that helps people with disabilities; technology will be everywhere in our daily lives, even more so than it is in the present day. Technology is vital for humans to evolve and progress society, which is why the young adults of generation z should become accustomed to technology and use it to their advantage rather than being against it. I would encourage everyone to become more educated in technology and choose a technology-oriented career path; if everyone is well versed in using computers or machinery, the transition into a tech filled world will be much smoother, and less people will suffer as a result of not being familiar with technology. We cannot stop the evolution of technology, but we can adapt to it and use it to better our lives.
Whether it will make you rich and prosperous or make you lose your job, technology will surely affect the future careers of everyone in our society; the decision you make in the near future could determine how technology impacts your life in the next 20 years.
Sources: https://www.businessinsider.com/10-ways-technology-will-change-the-world-by-2025-2014-6
Week 17- Chapter 6: Trigonometry
The focus of this week is Trigonometry.
Important terms:
Initial Arm: The arm of an angle that lies on the x-axis.
Standard position: When the initial arm is on the positive x-axis.
Terminal arm: The terminal arm is the other arm, it can be anywhere and it determines the angle.
Refrence angle: The closest angle between the Terminal arm and the x-axis.
Coterminal: Standard angle that shares the same terminal arm as another angle.
Rotation Angle: The angle from the initial arm to the terminal arm.
Ex: 
Sine Law:
The sine law can be used to find an angle or length of a side. To find the length of a side use the top formula, to find an angle, use the bottom formula (the reciprocal). You can only use the sine law if you have both the angle and side of one of the fractions, such as angle A and side a, or angle B and side b, etc.
Cosine Law:
The cosine law can be used instead of the sine law, if there are no fractions that have a value for both the angle and the length of the side.
You can tell if you need to use sine law or cosine Law just by looking at a triangle. If there is an angle in the triangle with a side value across from it, you can use sine law. But, if the angles don’t have a side measurement across from them, you will have to use cosine law.
In this example, you can use sine law:
In this example, you have to use cosine law:
The CAST rule:
The cast rule is an easy way to remember, which trigometric ratios are positive and which are negative in every quadrant.
Updated SOH CAH TOA:
In this unit SOH CAH TOA is updated to SYR CXR and TYX. When given a (x,y) point on the terminal arm, we can determine the refrence angle using the updated SOH CAH TOA.
Week 16- Problem Solving Rational Expressions Involving Proportions
How to solve problems involving proportions:
How much lemon juice should be added to 9L water to make a lemonade that is 30% lemon juice?
Step one: Make an equation by reading the question and identifying the components of the equation.
Step two: Simplify the equation by cross-multiplying and moving all the X’s to one side.
Step three: Solve the equation by isolating the X. The answer is 27/7 Litres.
Week 14- Multiplying and Dividing Rational Expressions
Multiplying rational expressions requires three steps:
Step one: Factor the expression
Step two: determine the non permissible values. Non-permissible values are numbers that result in a zero in the denominator.
Step three: cancel out the common terms, and you get your answer.
Week 13: Graphing Linead Reciprocal Functions
This week I learned about graphing reciprocal functions.
Step 1: Graph the linear function:
Step 2: Identify the asymptotes by first identifying the invariant points (the invariant points are always at the 1 and -1 y-axis points on the linear function, the asymptote goes directly through the middle of the invariant points, the other asymptote is always on the x-axis).
Step 3: Graph the hyperbolas:
Week 12: Solving Quadratic Systems of Equations
This week I learned about solving quadratic systems of equations.
A linear-quadratic system can have 3 possible solutions:
1) 2 solutions (line intersects parabola at 2 points)
2) 1 solution (Line intersects parabola at 1 point)
3) 0 solutions (line doesn’t intersect parabola)
A quadratic-quadratic system could have 4 possible solutions:
1) 2 solutions (parabolas intersect at 2 points)
2) 1 solution (parabolas intersect at 1 point)
3) 0 solutions (parabolas don’t intersect)
4) infinite solutions (parabolas are on top of one another)
How to solve a quadratic-quadratic system graphically:
Step one: Identify clues from the equations
Step two: graph the equations
Step three: Identify the intersects
The parabolas have 2 solutions (-2,-2) and (2,-2)
Week 11- Solving Quadratic Inequalities
This week in math I learned about solving quadratic inequalities.
When solving quadratic inequalities, you always have to remember that if you are dividing by a negative, you have to flip the inequality sign.
Step 1: Find the roots of a quadratic inequality:
Step 2: Insert the roots into a numbe line:
Then you can pick 3 numbers from the 3 sections on the number line and insert them into the original equation.
I picked 0 becuase it is quick and easy:
I got a positive number so, any number below 2 is positive.
Then I did the same with the number 3:
I got a negative number, that means that all numbers between 2 and 7 would result in a negative number.
Then I did the same with 10 and got a positive number, which means all numbers above 7 would result in a positive number. But the equation is asking which number could be inserted into the equation that would result in negative number. Looking back at the number line I see that all numbers beteeen 2 and 7 would result in a negative number. So the answer is 2<x<7.
Week 10- Sequences and Series Review
The unit I needed to recall the most was the Sequences and Series unit. Specifically Infinite Geometric Series. These are the types of infinite geometric series:
Diverging series expand each time so its impossible to determine a sum because the numbers grow so much each time they are multipled by the common ratio. In a diverging series the common ratio has to be negative.
Unlike diverging series, converging series do have a sum, converging series converge, and eventually the numbers will be so small that you could determine a sum. The ratio for a converging series needs to be bigger than -1 and smaller than 0.
This is the formula for finding the sum of a converging series .