Category: Math 11

In week 5 of Pre-Calculus 12, we learned how to factor. We learned about Factoring 1-2-3

Factoring 1-2-3 consists of 3 steps which are the following

Step 1. Find one thing in common. Remove the greatest common factor. In this case, everything was divisible by 2 so we can factor that out and put that as a coefficient.

Step 2. 2 terms? Difference of squares. 4 is a perfect square and 16 is so we can factor those out and simplify it as seen in the photo below. We should know that (2x)(2x) will leave us with $latex 4x^2

Step 3. 3 Terms? Is there a pattern? This example, we want to follow the rules, product of C, sum of B with the equation being (a+b+c). So we find the factors of C(12)that will add up to B(8). In this case, 2×6 equals C and 2+6 equals to B so that will be part of our final answer. And we should already know that x multiplied by x will leave us with $latex x^2 so our final answer will be (x+2)(x+6).

If you get stuck at a step, then it means that it is prime and not factorable. Below is an example. After we went through step 1, we couldn’t factor out anything else.

This week in Pre-Calculus 11, I learned how to multiply radicals with the same indexes.

I’ll be showing you how to multiply mixed radicals and entire radicals with the same indexes.

So first, for entire radicals, we simply multiply the radicals together and put it back under a square root. The index does not get changed at all as seen below.

We must then simplify the radical if possible as seen below.

Next, we have entire radicals, we basically follow the same rules as above (multiplying the radicand then simplifying), but this time we multiply the coefficients as well as seen below.

We then simplify if needed. In our case we needed to as seen below.

 

 

This week in Precalc 11, we covered addition and subtraction of radicals

I learned that when we want to add or subtract 2 radicals, the radicand has to be the same.

The rules about adding and subtracting radicals are

The radicands and indexes must be the same, when we add or subtract, the radicand stays the same but the coefficient changes.

For example: This equation has the same radicand so we can simply just add the coefficients together. We DO NOT touch the index or radicand.

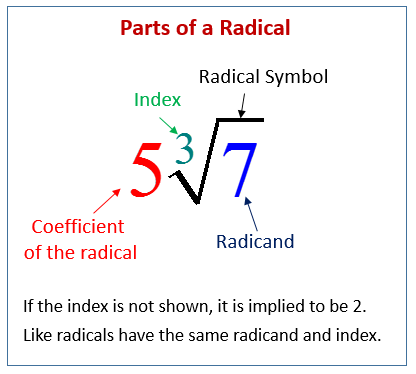
The radicands are the same so we can add them as seen below

 

 

Next question

When we want to add 2 radicals that are not simplified and in entire form with different radicands, we first simplify the radicand and turn it into a mixed radical. We then can add the 2 radicals if they have the same radicand. Here are the steps in picture.

For subtraction, we follow the same steps until the end. Instead of adding the coefficients, we subtract them.

Sometimes the radicands won’t end up being the same after we simplify them so the mixed radicands would be our final answer as seen below.

The bottom equation is our final answer.

This week in Precalculus 11, I learned about the exponent laws. I learned the following:

Anything with the exponent of 0 is equal to 1 because X has an invisible coefficient of 1 in front of the variable.

I also learned when multiplying numbers that have coefficients, we multiply the whole number if applicable then add the exponents together.

For an example: 2y^5 x 3y^4 we would first multiply 2 and 3 together then add the exponent (5,4) and we end up with 6y^9

When you have dont have brackets, the exponent is lazy and will only apply to the closest number. eg  -2^4, the exponent 4 only gets applied too the 2 and not the negative so the answer would be -16 but if we had brackets, we would have (-2)^4 and would end up with 16 because the exponent has to apply to everything in the brackets.

When you have negative exponent like 3^-1 you can put it in a fraction by simply putting a 1 over top and end up with 1/3^1 because the exponent becomes opposite (+/-)

When dividing numbers with coefficients, we take the difference of the exponents.

EG. 4y^5 ÷ 2y^9 so we take the coefficents and divide them (4÷2=2) and the difference between exponent 5,9 is 4 so that will be in our final answer of 2y^4

 

 

This week, I learned about exponents and radicals. I chose this topic because I was able to understand it pretty quickly and it is easy to remember. I learned that there are numbers at the end of the radicand called the “index” which tells you what the root number is and if there is no number it means that it is a square root (^2).

For example if the index is a 2 or no number it would be suare root and if 3 it would be a cube root, and so on.

This would be 3 because 3×3=9

 

 

This would be 3 as well because 3×3 =9 and 9×3=27 for a total of 3times itself 3 times.

I also learned that there can be coefficients beside the radical that is a multiplier.

For example, if it’s asking for the square root of 49 and there is a coefficient (3) beside it, you would find the square root which is 7 and multiply it by the coefficient, in my example,(3).