With this being the last blog post of Precalculus 11, I want to talk about 5 things I learned in this class that have been enjoyable or eye-opening.
Sine law & cosine law
- these 2 laws have to be one of the most interesting things I learned this year because they took everything that I learned in Math 10 and summed it up into formulas. This is way easier to use than SOH CAH TOA or anything similar.
- here are the formulas
- Sine Law: Useful for finding unknown sides or angles in non-right triangles when we know either:
- Two angles and one side
- Two sides and a non-included angle
- Cosine Law: Useful for solving triangles when we know:
- Two sides and the included angle
- All three sides to find any angle
Quadratic formula
- this formula is another example of a formula that I could’ve used in math 10 to make math a little easier. once you have the formula memorized it becomes a straightforward process to apply it to any quadratic equation and compared to other factoring methods like completing the square or grouping, this is way more straight forward.
- here is the formula


- once you have identified your values and put them in the formula you start solving for x
- by only solving the discriminant
 you can find the nature of the roots
you can find the nature of the roots - if discriminant = 0 —- there is 1 solution
- if discriminant = negative —– no solution
- if discriminant = positive —– 2 solutions
Radicals as powers (flower power)
- viewing radicals as fractional exponents makes working with them a lot easier. once you apply the radical as a power you can easily apply all the exponent rules to the expression.
- the denominator is the root.
- the numerator is the power

Graphing quadratic functions
- graphing quadratic functions provides a visual representation of the relationships between variables. it shows how many units the parent functions needs to move around.
- The graph of a quadratic function is a parabola, which is a U-shaped curve. The direction and openness of the parabola depend on the sign of the coefficients. If a > 0, the parabola opens upwards, and if 0>a the parabola opens downwards.
- Vertical Translations: Adding a number moves the graph up. Subtracting a number moves the graph down.
- Horizontal Translations: Adding a number inside the function shifts the graph left. Subtracting a number inside the function shifts the graph right.
- Vertical Stretching/Compression: Multiplying the function by a number: If the number is greater than 1, it stretches the graph vertically. If the number is between 0 and 1, it compresses the graph vertically
Restrictions with radical
- the last thing is the restrictions that come with radicals and even fractions. restrictions ensure that we have real number solutions and that the solution is not undefined/no solution.
- Even-Root Radicals (e.g., Square Roots):
- The radicand (the number under the root) must be non-negative. This is because the square root of a negative number is not defined in the real number system (it belongs to the complex number system).
- For example, square root of x, .
- Odd-Root Radicals (e.g., Cube Roots):
- The radicand can be any real number (positive, negative, or zero). This is because the cube root of a negative number is a negative number, and the cube root of a positive number is a positive number.
- For example, cube root of x, all real x.






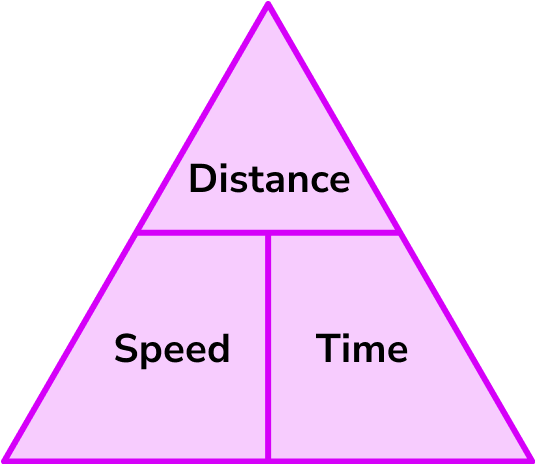 if you want to find speed, you cover speed with your finger and see that distance is on top of time, this means that we need to divide distance over time to figure out speed. The same goes for the rest.
if you want to find speed, you cover speed with your finger and see that distance is on top of time, this means that we need to divide distance over time to figure out speed. The same goes for the rest.

















