Week 7 – Interpreting the Discriminant

Posted on March 18, 2018 by shannonf2015

Last week, we have learned how to determine the number of solutions of a quadratic equation without solving the equation. To be able to do that, we look at the discriminant!

The discriminant is the radicand of the quadratic formula: $b^2-4ac$

This is how to determine the number of solutions by the discriminant.

Two real roots when $b^2-4ac$ > 0

If the discriminant is positive (so greater than 0), it has two solutions. (It touches the x-axis twice)

Exactly one real root when $b^2-4ac$ = 0

If the discriminant is zero, that means there is exactly one solution (Just touches the x-axis once)

No real roots when $b^2-4ac$ < 0

If the discriminant is a negative number (so smaller than 0), it has no solutions (Does not touch the x-axis)

Now that we understand how to use the discriminant to determine the number of solutions, let’s apply these to some equations.

First example: Calculate the value of the discriminant and determine how many solutions.

$4x^2+2x+3=0$

Remember the quadratic equation is : $ax^2+bx+c=0$

Therefore: a = 4, b = 2, c = 3

Plug in the numbers to determine the discriminant.

$b^2-4ac$

$2^2-4(4)(3)$

4-16(3)

4-48

-44

Since the discriminant is negative, it has no solutions; no real roots.

Now let’s look at another example where we have to create an equation with a given number of roots.

Determine the values of y of the equation which has two real roots.

$5x^2+3x+y=0$

To have two real roots, the value of its discriminant must be greater than 0.

So we use: $b^2-4ac$ > 0

Substitute: a = 5, b = 3, and c = y

$3^2-4(5)(y)$ > 0

Simplify.

9 – 20y > 0

Move the 9 to the other side to isolate the variable.

-20y > -9

Divide both sides by -20.

y > $\frac{9}{20}$

Since we divided both sides by a negative number, we must switch the inequality symbol.

y < $\frac{9}{20}$

Opinion Piece – Should Teams use First Nations as Mascots or Logos?

Posted on March 14, 2018 by shannonf2015

First Nations for Sports

https://canadianhistoryworkshop.files.wordpress.com/2013/01/treaty-8-logo_fliped.jpg

Sports teams have used First Nations as mascots and logos for many years, causing controversy on whether the act should continue or be prohibited. Some indigenous people find the team logos offensive and unnecessary, while others, including fans of the popular sports teams and First Nations themselves, believe it is not derogatory towards First Nations.

There are positive influences in using First Nation culture as a logo. For one thing, it brings awareness to those who are blinded to notice the presence and history of First Nations. It carries their footprints through a well-known brand, giving them recognition for being the owners of the land. While this may seem like a positive trend, there is still controversy on certain team logos and mascots, which dehumanizes them with exaggerated features or stereotypical images. This demeans the Aboriginal people resulting in them being placed into an inferior category; therefore, there needs to be a limitation. Having said that, referring to them as First Nations does not demean them. For these reasons, sports teams should be allowed to use First Nations as their logo, provided they seek their permission and approval. Take Edmonton Eskimos as an example. The term “Eskimo” referred to the Inuit people as eaters of raw meat; in other words, unintelligent cavemen. The Inuit community have spoken out hoping for a name change, but no change was made. Despite having the federal government and Inuit organizations stop using the word in early 1970s, the Edmonton CFO football team continue to use it. Words used to appropriate a culture and turning them into entertainment is disrespectful. In this case, the Edmonton Eskimos should have considered the Inuit people and communicated with them first to avoid causing harm.
Using First Nations as logos should not be banned, but there are certain boundaries that need to be considered. Rita Pyrillis, a Native American born and raised in Chicago, has shared her story of the difficulty living with transparency through her writing Sorry for not being a Stereotype. People assume she is an immigrant as her appearance does not fit the perception of a First Nation to others surrounding her. They see right through her, unable to tell what is fact or what is fiction: “Sometimes strangers think I’m from another time. They wonder if I live in a teepee or make my own buckskin clothes or have ever hunted buffalo.” (Pyrillis). Based on these stereotypes, they are treated as simple people stuck in the past. To this extent, sports teams should research the history behind the names they plan to use to avoid generalizing an image of First Nations through their logo. In the graphic novel Rising Above by Steven Keewatin Sanderson, he shares examples of positive and negative stereotypes that exists throughout accessible resources. Positive stereotypes deconstruct First Nations, adding new perceptions to their image, which both raises the standards and gives an unrealistic image. Negative stereotypes generalize their actions and appearance, giving an identity that small minded people would understand. Steven, using the example of Pocahontas, wrote about the fact that many illustrated novels sexualize First Nations, portraying them as weak and nothing more: “Aboriginal women are more than just objects to be sexualized, they are strong powerful leaders and the givers of life.” (Sanderson, 39). In view of the above, sports teams should not deconstruct First Nations to meet their standards for logos. They are acceptable as long as they keep their logos realistic, respecting and honouring First Nations true identities.

In conclusion, it is acceptable for sports teams to use First Nations as their logos while being mindful and respectful of the symbolic meanings and significance to the First Nations. Researching about their history and communicating with the owners of the culture is far more acceptable than just borrowing from it.

Works Cited

Obed, Natan. Edmonton Eskimos Name Debate. 22 November 2017. March 2018. <https://globalnews.ca/video/3874931/edmonton-eskimos-name-debate>.

Snowdon, Wallis. Edmonton Eskimos name an insult to the Inuit, says local Inuk woman. 14 November 2017 . Monday March 2018 . <http://www.cbc.ca/news/canada/edmonton/edmonton-eskimos-name-change-inuk-critic-1.4401422 >.

Week 6 – Solving Quadratic Equations

Posted on March 10, 2018 by shannonf2015

This week, we have learned how to solve quadratic equations by completing the square and applying the quadratic formula when the equation is not factorable.

A quadratic equation includes at least one squared variable and has the following form: ax² + bx + c = 0

a, b and c are constants or numerical coefficients and x is the unknown variable.

Let’s start with the method of completing the squares. This method is quite a long process, it depends on the equation. The best would be to see if it is factorable, if it is not or factoring is too difficult for the equation, you can use the completing the squares method or using the quadratic formula.

Completing the Squares

Lets start with an example:

${x^2}+{4x}=2$

To be able to solve this equation, it must equal to zero as it is a quadratic equation. In this example, we have to move the 2 to the other side so that it becomes zero:

${x^2}+{4x}-2=0$

It becomes -2 because when you move it to the other side of the equation, you switch the signs.

Now that we have it written in the proper equation for us to solve, first check if it is factorable. As there are no numbers that both multiply to equal to two and add to equal 4 (only option is $2\times{1}$ ). So we must use either the completing the squares or formula. In this example, I will be using the completing the squares method.

First, we make two places that are both + and – to get zero pairs in between the inner term and the constant:

Then we find what is the missing constant. To do that, we divide the inner term in two and square it. The answer will be 4. Then we factor for those three terms.

Now that you got ${(x + 2)}^2$ , write the other numbers that are next to it: -4 -2 which equals to -6.

Isolate the variable by moving -6 to the other side of the equation. Then square both sides to get x + 2 = $\sqrt{6}$ . Make sure to include both + and – signs next to the radical because it has two options.

Then move the 2 to the other side of the equation to isolate x.

x = -2 ± $\sqrt{6}$

Now let’s see how we can apply the quadratic formula.

${2x}^2-2x-1=0$

This is the same form as:

${ax}^2+bx+c=0$

a=2, b=-2, c=-1

Formula:

Substitute the variables with the numbers given.

Once you substitute it, simplify it while making sure to keep the + and – sign next to the radical.

Week 5 – Factoring Polynomials

Posted on March 3, 2018March 4, 2018 by shannonf2015

I haven’t really learned much starting this week, but I have learned quite interesting new techniques on factoring polynomials.

Let’s start off with this acronym I have learned to help with the process.

CDPEU – Can Divers Pee Easily Underwater?

Common

Difference of Squares

Pattern

Easy

Ugly

Let’s breakdown what each term means before getting into the factoring process.

Common means find what is common between the terms, the GCF (Greatest common factor).
Difference of Squares means if there are two binomials and they are subtracting.
Pattern means if they have three terms following the pattern: $x^2$ $x$ #
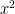
Easy means the easy ones to factor out (straightforward) : $x^2$
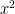
Ugly ones are the polynomials that not so straightforward and need more work. We’ll see what method we can use later on. : $a{x^2}$

Now let’s apply these steps!

To start, refer to the list above we just talked about.

The first step is find what is common. To do so, write all the possible factors of both coefficients and see what variables both terms have in common.

After we found all possible factors of 49 and 14, we can see that the common number is 7. Both terms also have at least one x variable. Therefore, you would factor out 7x :

Write out the rest of the values in the bracket next to 7x, making sure when you multiply the values in the bracket with 7x, it will give you the same original expression.

Do we stop here? Well let’s check the list.

Difference of squares is the next one. To tell if it’s a difference of squares, it must have two terms and also be able to form conjugates (subtraction of two terms).

In our example, (7x – 2) does not have an 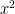$x^2$ , so we cannot factor them out any further. Therefore, it is not a difference of squares.

Next step is pattern. Well this doesn’t work because it doesn’t have three terms, therefore, we cannot simplify it any further.

It is therefore : 7x(7x – 2)

Now let’s look at an example with three terms.

First step is looking for something in common. As we can see, there’s nothing in common so let’s go to the next step.

Second, is it a difference of squares? No! There is three terms.

So, thirdly, let’s check if there’s the pattern : 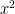$x^2$ $x$ #

Yes!

The first thing to do is draw two brackets and start with putting in x to get 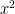$x^2$ . Then factor out all the possibilities for 24.

To decide which two numbers we must use for 24, the two numbers that are multiplying must equal to 10 (the inner term) when added together. So the pair to choose would be 6×4. The sign depends on the inner term. It’s positive and so is 24 so therefore we use ++.

This is the final expression factored.

(x + 4)(x + 6)

To check if it’s correct, we can foil back in the values, meaning distribute all the numbers back by multiplying.

So far, these are both easy. But let’s get into the uglier ones.

There are no common factors here, and there are no differences of squares. There is the pattern though with the three terms, but this one seems a little more complicated than the other one we just did.

A method I learned for these ugly trinomials is using the box.

You split a box into four sections, writing in first the value with 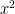$x^2$ which in this example is $25{x^2}$ on the top left corner and the constant on the bottom right corner.