Graphing linear inequalities is building off of graphing linear equations. They are graphed the same way, the number before x represents the slope(rise over run) and the second term shows the y-intercept.
The only difference is that when graphed, there is going to be a section shaded. This section represents all the numbers that satisfy the equation. If the line is dotted, those numbers are not included and will be represented by <>. If the line is solid, those numbers will also satisfy the equation and the inequality sign will have an equal on it ≤≥.
To find what the inequality is if the graph is already given.
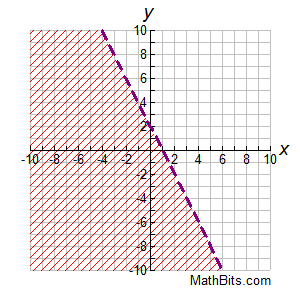
y _ -2x + 2
You create the equation using y = mx + b or slope y-intercept form.
Now, we can take a number in the shaded area and make a true statement.
(0,0) is a coordinate in the shaded area.
0 _ -2(0) +2
0 _ 2
Since 2 is greater than 0 and the line is dotted, we know we need < to make the statement true
0 < 2
We can go back and add < to the blank space of our equation
y < -2x + 2
If we are given the equation to graph, we follow similar steps.
3x + 6 ≤ 2y
Divide by 2 to bring the equation in slope y-intercept form
3⁄2x+3 ≤ y
Once graphed, you can choose any coordinate in one of the sides, (5,0)
3⁄2(5)+3 ≤ 0
15⁄2 + 6⁄2 ≤ 0
21⁄2 ≤ 0
So when graphing, we know to show a solid line because of the inequality symbol and to shade in the side that includes the coordinate that satisfies the equation.