Archive of ‘Math 11’ category
This week in pre calc we reviewed the idea of trigonometry and then learned new things to add on to what we already knew.
So one of the things that we learned was the SIN LAW.
The sin law is

The lower case letters are the side length measurements and the upper case letters are the angles degrees.
Because the Sine Law works with the angles of triangles and the measurements of the triangles side then it’s useful in finding a missing angle or side.
how to use the sin law:

step 1: fill in what you know from the image
sinA/a = sinB/b = sinC/c
sinA/5 = Sin80/7 =sinC/c
and then u would take the two that has the most information/ what your looking for
in this case it would be sinA/5 = sin80/7
because were trying to find angle A
so we would next solve the equation
sinA = 5sin80/7
A=sin-1 (5sin80/7)
A= 44.7
and now you know what angle A is.
This week in PreCalc 11 we learned how to solve rational equations.
Rational equations are equations containing at least one fraction whose numerator or denominator is a variable.
There are two ways to solve rational equations, one of them is multiplying every term by each of the denominators or cross multiplication. Cross multiplication only works when there are two fractions and one is on each side of the equal sign (remember that a single number for example 4 is a fraction still because its over 1) I like multiplying the denominator better because multiplying by the denominator is a strategy that will work with every type of rational equation.
Example:
step 1: factor
step 2: multiply by the denominator
step 3: Non permissible values
step 4: solve
ex: (there are no non- P values because there aren’t any variables in the denominator)

This week in PreCalc 11 we revisited the idea of rational expressions and learned how to add together rational expressions with variables in the denominator.
for example:
when we add the rational expressions together the denominator needs to be the same so we take a common factor of the two denominators.
And we always need to remember that the denominator connot equal 0. So if the denominator is 8x x cannot equal 0. And if it is 8 + x, x cannot equal -8.
example:
6/5x + 4/3x
we would find the common factor of both denominators which in this case is 15 so we would multiply the top and bottom so the bottom equals 15
6/5x + 4/3x -> 18/15x + 20/15x
and then we could add them together
18+20/15x
38/15x is your answer
and if you could from this step you could simplify this expressions. (notice there isn’t an equal sign because expressions don’t contain equal signs)
last we need to write the restrictions for x so in this case x cannot equal 0.
This week in pre calc 11 we learned how to determine the difference between and absolute value graph and reciprocal value graph.
we know that an absolute value graph cannot have any negatives at all because when a number is in the absolute value symbol even if it is negative it must change to positive, that’s one way we can tell it is an absolute value graph, another way is that it will make a V shape if it is linear
ex: 
and if it’s a quadratic function graph it will make a W shape
ex: 
that is unless it is a horizontal line.
To tell if it is a reciprical you will notice that it will go in the negatives in most cases
ex: 
ex: 
This week in PreCalc 11,
we learned how to graph absolute value functions.
An Absolute Value Functions is a function that has an expression within absolute value symbols. We were taught before that Absolute Values, was when the number is between the absolute value symbols must come out a positive. Example, | -2 | = 2 .
Example:
y = | -2x + 4 |
Step 1: Graph the Parent Function
The first step is to graph the parent function. The parent function is the same function but without the absolute value symbols.
In this case, the parent function is y = -2x + 4.

Step 2: change the Negative Values to positive
y-values in the parent function can’t be negative that’s why there’s an absolute value function. So all we have to do is change the negative y-values into a positive and then graph it again.

and as u can see the parent function is shown in this graph but where it intercepts with the x intercept is where it bounces back up.
This week in Pre Calc 11,
We learned how to solve systems of equations using substitution.
A way to solve a linear system or quadratic system or both is to use the substitution method.
We do this by substituting a y-value in an equation with the other. to start you first substitute y in the second equation with the first equation (since y = y). Because it is harder to solve an equation if it has two variables. After substituting y into the equation and solving for x, the value of x can then be used to find y by substituting the number you found, with x. While using the substitution method, you can also start by substituting x in the second equation with the first equation. You can start of by substituting x first or y your choice.
For example :
y = 2x + 2
y = x^2 + 5x +4
Take the first equation and replace it with the y in the second.
2x + 2 = x^2 + 5x +4
Solve for x.
0= x^2+3x+2
0= (x+3)(x+1)
This week was a shortened week so we didn’t go over as much stuff as the previous weeks.
We learned how to graph quadratic and linear inequalities in one variable and two variables.
To graph a quadratic inequality you need to find the y- intercept and the vertex and use the clues given to graph it and find possible solutions and where to shade in the graph.
For example:
y>x^2+6x-18
the y intercept is -18
you can tell it opens up because the x^2 is positive
the vertex moved
and the pattern is 1.3.5 because the leading coefficient is 1
So then you would factor
y>x^2+6x-18
y> (x+8)(x-2)
and with those you can find your vertex from -8 and +2 being your x- intercepts
to find the vertex you would do -8+2/2
so your vertex is -3
and by plugging it back into you equation you can find the minimum point connected to the vertex and graph it
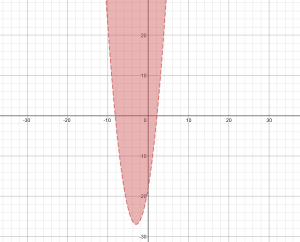
we know that its a doted line because the > doesn’t have a line underneath it
and we can put a test point into the original equation to know if its shaded in, in the certain area.
PreCalc 11
We did review for most of the week so this is one of the things i reviewed today. so i revisited the idea of factoring polynomial expressions like we learned last year in grade 10, and like we learned in the previous units.
For example, when we started the practicing of factoring expressions I remembered that for the second number in the trinomial (abc: letter B) it was the sum of adding the two numbers together and the third number in the trinomial (abc: letter C) was the sum of the two numbers multiplied.
For example:
x2 + 7x + 10
I could go through the list of factors if 10 and then find 2 that add together to get the sum of 7.
1-10
2-5
you notice that 2+5 equals 10 so then you input those into the expression
(x + 2) (x + 5)
This week we continued to go over quadratic functions.
I learned that you could model problems with quadratic equations,
for example: Find two integers with a sum of 24 and the greatest possible product.
x+y= 2
product is a multiplication word so we know that we have to multiply it to find it meaning that the PRODUCT= x ∙ y
we have to re-arrange the equation that we already have so that we can get it to have only one variable.
y= 24-x
product=x ∙ (24-x)
(zero product law)
x=0 (1st term)
x=24 (inside brackets)
LOS= 0+24 divided by 2
=12
then we would insert that into our standard form
p(x)= x (24-x)
p(12)= 12 (24-12)
=144
144 is our maximum product
I found it very interesting that we didn’t need to have a word problem that involves something being thrown or going up nd down like a U shape and that instead we could still help solve it and graph it like one.
This week was a tough week coming back from spring break and my most struggle this week was trying to stay in focus the whole class since we had two weeks off I definitely missed a lot this week because I couldn’t keep in focus, we learned how to graph quadratic equations,
we learned that the vertex is the exact midpoint of the x- intercepts.
the standard form is ax² + bx + c = 0
So for a function to be quadratic it has to have x2 in it, so that it will graph a parabola that will make a U shape on the graph.
The parent function or where all parabolas start is From this form y=x2
we can change the equation so that we can add variables to show if the parabola will be moved up or down, side to side, which way it faces and how skinny or thick it will be.
For example:
y=x2+5
this means the parabola will be 5 up on the y-axis but zero on the x-axis.
7 key characteristics: Vertex (most important), Domain, Range, Maximum/Minimum, x-intercept, y-intercept, and the line of symmetry.









