January 16, 2019

What I learned in Grade 9 Surface Area

Let’s start off with Surface Area. Surface area is the combined measurement of all faces in a 3D shape. This usually applies to prisms, prisms are solid objects that have identical ends and flat faces.

Many different prisms have different formulas to solve them, but boiled down it’s simply finding the area of each face and combining them together.

Composite surface area isn’t very different either, composite surface area is when two prisms are glued together to have a sort of “super-prism”, solving the surface area is similar but with a twist.

Let’s say these are the dimensions for our composite shape:

Rectangular Prism 1:

Length : 15mm

Height :8mm

Width : 10mm

Rectangular Prism 2:

Length :6mm

Height: 4mm

Width : 5mm

In order to find the surface area of a composite prism, you first need to find the surface area of both prisms

Rectangular Prism 1: 700mm

Rectangular Prism 2: 148mm

Let’s say Prism 2 is on top of Prism 1, and the side glued to Prism 1 is 30mm, since its already glued to Prism 1, then it’s practically vanished away from both prisms, so we have to subtract 30mm x2, one for the 30mm taken away from Prism 1, and again for Prism 2.

So we add together both surface areas

848mm

And subtract the 60mm

788mm

And there we go, that’s how you solve for composite surface area, the same process applies to different shapes, it doesn’t matter as long as you solve for the surface area and the side that’s attached to the bigger shape

December 13, 2018

What I learned in Grade 9 Polynomials

So, let’s start with the low-hanging fruit. What is a polynomial? For starters, a Polynomial is a sentence for algebraic equations, something like this:

4xy+3x + 2x+2

It’s usually comprised of numbers, variables and exponents. A set of these is called a term, terms can help us identify what kind of algebraic equation it is.

3xy is a Monomial

5ab-3a is a Binomial

4vk-3k+v is a Trinomial

5cd-3x+4-y-12 is a Polynomial

Note that anything that has 4 or more terms is classified as a Polynomial.

Polynomials also have degrees, in simple terms the degree of a Polynomial is the largest exponent the Polynomial has.

( $6x^3$ ) + ( $6xy^2$ ) – ( $3x$ ) + ( $2$ )

3 3 1 0

If there are two different variables, add them together to get the degree

If two exponents are both the largest, then the Degree is still the same.

Operating with Polynomials

Addition :

Adding Polynomials is as simple as it can get, add all like terms together to get the answer.

( $4x^2$ ) + ( $2x^2$ ) = $6x^2$

Subtraction:

Subtracting Polynomials only requires you to flip anything to the right of the minus symbol, all negatives become positives and all positives become negative, afterwards add them as you would normally.

( $3xy^2$ ) – ( $4xy^2$ + 3)

( $3xy^2$ ) + ( $-4xy^2$ -3) =

( $-xy^2$ )

Multiplication:

Multiplying is quite easy, multiply all like terms together, but add the exponents up.

( $3a^3b^3\cdot 5a^2b$ ) =

$15a^5b^4$

Division:

Like multiplication, all you have to do is divide all like terms together, but subtract the exponents instead.

( $10x^3y^2 \div 5x^2y$ )=

$2xy$

A few tips I could share:

If there is a number outside of the bracket, then you need to distribute the number to everything inside the bracket

Likewise, if there’s an operation behind the number closest to the bracket, leave it as is and distribute the number to everything inside the bracket.

If you’re dealing with smaller polynomials, you can use algebra tiles.

Have $x^2$ as a large square

$x$ as a rectangle

and 1 as a small square

Color them in on one side

Flip them over to the clear side when using a negative

Use them as you like.

December 10, 2018

Narrative Mood

The mood of the poem is dramatic, intense and extremely satirical. It makes fun of modern tropes like Fortnite and 1v1 duels, and it’s supposed to be an exaggerated interpretation of how a modern showdown would happen with satire themes. It primarily makes fun of the Fortnite community, since it’s supposedly filled only with young, immature and oblivious children, it also makes fun of how an honourable duel would go nowadays, instead of a chivalrous and respectable battle, it goes down into a stalemate with both arguing as to who won in the end.

We chose our pictures to show how the duel would go if it were nowadays, it has intentionally low production values with little props, we focused more on action and body language, and it’s supposed to have that “so bad it’s kind of good” feeling.

November 17, 2018

What I learned in Grade 9 Exponents

So, what have I learned about Exponents up until this point? Well, why don’t we start off with the basics.

What is an exponent?

An exponent is a simple version of multiplying a number to itself, the exponent is located at the top right of a variable, like so:

$x^2$

The exponent shows us how many times it has to multiply itself, if a number like 2 is raised to the power of 2, then the equation will look like (2 x 2), if it’s raised to the power of 4, then it’s (2 x 2 x 2 x 2).

There are special terms for certain exponents however, the power of 2 is called a square, the power of 3 is called a cube, the rest are simply “x raised to the power of y”

What is the difference between evaluating and simplifying?

If we had a question like “ $3^2\cdot 3^4$ “, then the end result can be “ $3^6$ ” without any repercussions, that is evaluating.

But if we had a question like “ $4^4\cdot 4^5$ “, then we need to simplify it to it’s lowest form, the end result is then “1280” instead of “ $4^9$ “, that is simplifying.

What is Multiplication Law?

If we are multiplying two exponents that have the same base, we can just add the exponents together.

$5^4\cdot 5^4$ = $5^8$

What is Division Law?

The opposite of multiplication law, if we are dividing two exponents that have the same base, we can just subtract the exponents.

$6^9\div 6^8$ = $6^1$

What is Power of a Power law?

If we have an exponent that has another exponent outside of it, then we need to multiply the exponents together.

( $7^3$ ) $^3$ = ( $7^9$ )

What are Exponents on Variables?

The only difference between exponents on numbers and exponents on variables is that there isn’t a simplified answer for exponents, since the value is unknown, then you can simply just add the exponents like in Multiplication law.

$x^2\cdot x^3$ = $x^5$

October 17, 2018

What I learned in Grade 9 Fractions

Here’s what I learned in Grade 9 fractions so far

For putting negative fractions on a number line, you have to determine the fraction’s value and put it on the number line

$\frac{1}{4}$ $-3\frac{3}{4}$

<5—-4 $-3\frac{3}{4}$ —3—-2—-1—-0 $\frac{1}{4}$ –1—-2—-3—-4—-5>

For $\frac{1}{4}$ , it’s clearly less than 1, but still has a value greater than zero, so it’s placed in between

For $-3\frac{3}{4}$ , since it’s a negative, it goes backwards on the number line, since if going up with positive means going on the right, negative means going on the left.

Comparing fractions is quite easy, it’s as simple as checking which one is bigger than the other, like this :

$\frac{1}{3}$ $\frac{2}{3}$

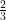
The line is clear as to which number is bigger, because 2 is clearly larger than 1. But since teachers want to test their students, you’re more likely going to find questions like these :

$\frac{1}{3}$ $\frac{3}{6}$

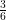
These questions aren’t as hard as you think either, all you need to do is make them equal, an easy way of doing this is by making sure both denominators are the same, and you can do this by multiplying.

$\frac{2}{6}$ $\frac{3}{6}$

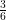
The reason why the 1 turned into 2 for the first number was because whatever happens to the denominator, happens to the numerator, it’s kind of like if 3 got caught stealing food from a grocery store, 1 would go down with them.

So now that both have equal denominators, both can easily be compared, you do this process with all fraction comparison questions that have uneven numerators and denominators.

Some numbers are a combination of a whole number and a fraction, like this :

$1\frac{1}{2}$

In order to make this into an improper fraction for use, we need to multiply the denominator to the whole number, then add the result to the numerator, the end result is something like this:

$\frac{3}{2}$

One important thing to do before comparing any fraction is to make all mixed into improper first for ease of solving.

For adding and subtracting fractions, it’s just as easy, you do the same steps, but with slightly different rules.

$\frac{1}{4}$ + $\frac{3}{12}$

So make both of them equal like so, make sure to multiply the numerator as well:

$\frac{3}{12}$ + $\frac{3}{12}$

= $\frac{6}{12}$

The same applies to subtraction questions as well. But when you put a negative, that’s when the whole thing flips on it’s head

$\frac{-6}{12}$ + $\frac{3}{12}$

$\frac{-8}{10}$ – $\frac{7}{10}$

Both addition and subtraction can catch you off guard if you’re not careful, if it’s an addition question like this, then do a little game that goes like this :

There are two nations, Positive and Negative, they are currently fighting a war of attrition, and every group of reinforcements can be decisive to winning a battle, there are multiple ways clever field commanders can strategize, some skirmishes can go like this:

Battle 1:

-6 negative soldiers vs. 3 positive soldiers

Whatever side is larger wins the battle, so the aftermath will look like this

-3 negative soldiers surviving

This is how some battles will play out, either side must win through sheer force of manpower. Another way the battle could play out is like this:

Battle 2

-8 negative infantry units vs. 7 positive infantry units

Since this is a subtraction question, or as commanders like to call a propaganda attack, all 7 positive units defect and aid the negative side:

-15 negative soldiers

This causes the Positive nation’s defenses to be overrun, if a subtraction question has a negative on the left side, then any positive must turn into a negative as well, negative + negative = negative.

Either nation would keep fighting, one may win the battle, but not the war, so the fight between Positive and Negative will continue until the end of time.

Multiplication and Division

All you have to do for Multiplication is direct and easy, just multiply straight across

$\frac{4}{7}\cdot\frac{6}{14}$

= $\frac{24}{98}$

And you’re done.

For Division, there are multiple ways you can go about dividing, but I’d say you should just use the Reciprocal method :

$\frac{12}{15}\div\frac{7}{9}$

Just flip the right fraction on it’s head then multiply

$\frac{12}{15}\cdot\frac{9}{7}$

= $\frac{108}{105}$

October 16, 2018

Hero Cultural Connections Infographic

October 1, 2018

Digital Footprint

How might your digital footprint affect your future opportunities? Give at least two examples.

If you attempt to enroll for a college or look for a job, your employers will sleuth the internet in search for any information that will shed light on your personality. They can check if you have any…undesirable traits like aggression, racism, or unprofessional behavior simply from any posts that you made in the past. While at face value these don’t give a 100% accurate reflection on your character, they will carefully scrutinize these since they have little to no information outside of your resume.

Any future relationships that you may have, like potential partners, might also check your profile to see if you have traits they may not want in someone they intend to spend their life with. If they find out that you are aggressive and abusive, they may turn you down and look for better people, besides, there are plenty more fish in the sea.

Describe at least three strategies that you can use to keep your digital footprint appropriate and safe.

Do not show any personal information. Use a username instead of your full name, don’t show your home address, keep your account so that only friends can see them, and overall be secure and keep any confidential information away from prying eyes.

Keep watch over your accounts and passwords. Always keep them in a security app like LastPass or Applock, and to reinforce security even further, make passwords for these security apps even harder than your personal account passwords

Don’t be reckless. By simply exercising caution you can effectively protect your information to a large degree.

What information did you learn that you would pass on to other students? How would you go about telling them?

Add the site name to the end of your password for certain sites, like using passwordInstagram, passwordSteam or passwordGmail

Be careful when dealing with location based services, since certain apps may request permission for services that aren’t even tied to their original use, like a Camera requesting location permission, while it has it’s fair share of positives, always be aware of the potential security threat.

There will always be people who would want to steal your personal information through viruses and spam mail, always make sure that your anti-virus is up to date and to be on the safe side, manually go through your emails and messages to make sure that no files like viruses are present.

Migo's Blog

My Riverside Rapid Digital Portfolio

Category Archives: Grade 9