surface area:

I found surface area was quite an important unit this year because I think it is important to learn how to calculate the size dimensions and measurements of a shape.

https://www.gigacalculator.com/calculators/surface-area-calculator.php

I learned how to find the surface area of this prism. What helped me the most was just writing all the combinations with these numbers.

• 8 x 12 (wxh)
• 12 x 5 (hxw)
• 5 x 8 (wxl)

8 x 12 = 96cm2 (192cm2)

12 x 5= 60cm2 (120cm2)

5 x 8=40cm2 (80cm2)

ADD: 392cm2

linear equations: 

I think that solving linear equations was a very important unit we learned this year. Being able to isolate the variable was something that I quite enjoyed.

ALWAYS REMEMBER

B= best//brackets

F=freinds//fractions

S=share//sort

D=deserts//divide

EX) 3x-2=12x+5

the first step we would need to do is to subtract 3x from each side *WHAT YOU DO TO ONE SIDE YOU DO TO THE OTHER*

the new equation:

-2=9x+5

based on BFSD we still do not have x by itself, meaning we still have to sort.

to get x on its own we need to subtract 5 from each side

NEW EQUATION:

-7=9x

the last step would be to divide 9 by 9 and 7 by 9

ANSWER:

-0.78

https://youtu.be/GmMX3-nTWbE

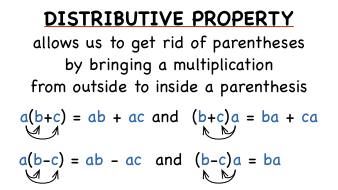
distributive properties 

I quite enjoyed the distributive law, I think it is important because it gives you a different approach on multiplying.

EX) 5(6x-12) + 2(x+3)

you would first need to multiply the 5 by the 6x (30x) and then the 5 multiplied by 12 (60)

and do the same on the opposite side…

if we were to re-wright the question it would now be…l

30x- 12 + 2x + 6

the next step would be to combine all the like terms.

ANSWER: 

32x +6

http://virtualnerd.com/sat-math/fundamentals/simplifying-expressions/distributive-property-definition

Similarity: 

I think learning about the different kinds of surveys are good to know.

here are the different kind of influences that can be put on a survey to make it bias, or not fair.

is the question bias– meaning does it show a preference towards a certain product or thing?

is the use of language easy to understand– is it clear and legible?

Ethics– does it report to an inappropriate behaviour/ against the law?

Cost– do the costs outweigh the benefits?

Time and timing– is it the appropriate time/ will the timing affect the results?

Privacy– does the person answering the questions feel like their answer will be kept confidential? Do they have the right to refuse to answer the survey?

Cultural sensitivity– do the questions affect the cultural groups/ will the question be offensive/ be aware/ do they have options?

Samples:

random sample: a random sample includes a systematic sample, which is unbias, and everyone has the same chance of winning. there is also a stratified sample meaning a certain amount of people get chosen randomly

random samples- show unbias results, and the response can be made to make predictions about the population.

non random samples

• convenience- only asking the people that walk in a certain hallway
• voluntary response sample- only those who wish to answer will, either have a very positive response or a very negative response.

Exponents: 

exponents were a very important unit, in my opinion, writing an exponent is so much easier than multiplying,

rules:

1. anything to the power of zero is 1
2. little numbers only multiply subtract add or divide by little numbers
3. the exponents tell you how many copies are being made
4. when it is a multiply question the exponents add
5. when it is a division question the exponents subtract.

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

 

Vocabulary:

Obtuse: More than 90 degrees, but less than 180

Right triangle: has one angle that is 90 degrees and a variety of other kinds of

Equilateral: means all the sides are the same and add up to 180 degrees, which would make each angle 60 degrees.

isosceles: has 2 of the same angles, and the same side lengths.

Scalene: means none of the sides are the same

Acute: has 3 acute angles

Similar: means that they have the same scale factor, or the same angles

Enlargement: means that the image is being made larger, so the numbers also need to be made larger EX) a diagram of a fly in a book, it is impossible that a fly can be the same size as a page in a book, meaning it is an enlargement

Reduction: means that the image is being made smaller. EX) Canada on a map, Canada can’t fit on a piece of paper, therefore it is a reduction.

 

How to find out if 2 triangles are similar

ALL ANGLES ARE EQUAL (CONGRUENT)

to find out if 2 triangles are similar is you can look at their angles, weather they are obtuse, if they have a right angle, and what their shape is.

the next thing to do is to make the ratio, but to make the ratio you need to decide which triangle is the image and which is the original.

I will be using the triangle on the left as the original and the triangle on the right is the image.

 

you need to make fractions to find the ratio- THE ORIGINAL ALWAYS GOES ON THE BOTTOM (make sure to line up the sides)

6/3   8/4   10/5

next divide-

6 ÷3= 2

8 ÷4=2

10 ÷5=2

in this case these 2 triangles are similar, and their scale factor is 2.

A faster way to solve an equation

if we use the example of the following ratios:

3/6   7/x   4/y

the way to solve it would be “the butterfly method”

where you would take the 3/6 and cross multiply it with the 7/x. which would equal 3x= 42.

next we need to divide 42 by 3 (14) which would be the solution for x

 

the next step would be to cross multiply 3/6 times 4/y which equals 3y=24

divide: y= 8

 

 

 

Linear Inequalities

Solving:

what do these signs mean?

(≥, ≤, >, <)

These signs represent the larger number(s) in the linear inequality, so if there is a 3x<5. this inequality would represent that 5 is larger than the 3x, another example is x≥5 this inequality represents that the x is more than or equal to 5

to solve a linear inequality you must know the correct order to do the steps, the order is BFSD, a good way to remember it is Best Friends Share Desserts, or the mathematical way Brackets Fractions Sort Divide

If we use the example of 3x+4 ≥ 12x -7 the first step would be to reduce the expression by subtracting or adding something to each side in order to get all of the x’s on one side and the numbers on the other (a lot like linear equations)

What we can do is we can take away 3x off of each x so now we are left with 4 ≥ 9x -7

as you can tell we still have to keep going because there are 2 number on one side of the sign and 1 on the other, and the whole point of these expressions is to find the value of x

the next step of this expression would be to add 7 to both of the coefficients to create yet another zero pair. Our expression is now 11 ≥ 9x. The last step would be to divide, and since the 9x is not a negative number, we do not have to flip the sign, you only do that what the x is negative.

9x/9=x  11/9= 1.2

but since this isn’t a pretty answer we could just leave it as 11≥9x

Graphing:

  

this image is showing that if the x is being poked, (like it is in image 1) that means that the line will be going to the left because the sign is opening up to the 11, not the x

but in the next example if the sign is poking the constant that means the arrow is going to the right because the x would be the bigger number.

“But when do I use a filled in do, or an open dot?”

You use the open dot when the sign does not have a small line under it, which means that the inequality is not including the number. You use a filled in dot when the sign has a small line under it, which means that that number is included in the inequality

Checking:

to check and see if you have done the inequality correctly is you replace the x in the inequality with your x equals number (answer) and you know you got the correct answer when the inequality is true based on the sign

 

 

 

images:

• https://www.varsitytutors.com/hotmath/hotmath_help/topics/graphing-inequalities-in-one-variable