Last week I explained how to multiply binomials, but now we will be multiplying with trinomials, with a larger amount of terms this can start to become confusing using FOIL, so I will show you how to break it down step by step. This has a few steps that you have to follow, however if you want to get the right answer its best to follow them at first, at least until you get the hang of the shortcuts. I have given you an example of an equation down below and I have gone through what all the steps in the photo. First you will FOIL but on each side of the subtraction side, making sure all the numbers get factored with each other once, next you will  you will combine like terms then you will multiply by the original co-efficient and the combine like terms again (terms with the same variables) then you will combine both halves of the equation.

4(a-4)(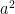-3a-6)-(4a-3)(4a+3)

This week week we started a new chapter; Polynomials. Polynomials are monomial or a sum or difference of monomials, they consist of terms normally separated by + or – signs. The hardest part about polynomials is probably remembering to combine like terms, like terms are terms that share the same exponents, and same variables. Down below I have showed you some examples of like terms, and in the photo you can see them in an equation.

Ex. – this would come out to be

When multiplying Binomials you use F(first term in each bracket) O(outside terms) I(inside terms) L(last term in each bracket)

This week brought us back to a thing as simple as volume and area, but only with a larger formula. The only difficult part about this is remembering the formulas, but other than that you just have to plug in your measurements. When referring to a pyramid we are referring to a right pyramid. The formulas we use for a pyramid is SA(Pyramid)= Area Base + Area of Triangular Faces -V(Pyramid)= 1/3 Abase(h)- SA(Cone)=πr² + πr – V(Cone)=1/3πr²h

And when you are plugging things into these formulas make sure you use your pie button rather then using 3.14, because otherwise your answer may be off.

This week we learned a lot of different methods, as their is a couple different systems that you can convert to and when converting within there is also a couple different methods too. I chose to show you a method of conversions within the metric system because the units are related to each other by factors of ten this allows us to use a method with a number line. It can be difficult to know when to use which methods and how to know when to use what method but the good thing about using the metric system is that we use it in our day to day life here in Canada so if the unit isn’t recognizable you can automatically rule out the fact that it will be a conversion within a single system, instead you will have to use the exact units (not normally whole numbers) to convert to the imperial system. Down below I have shown you how to use one method- the number line to convert from unit to another within the metric system.

In order to problem solve using these rations we first need to know when, and how to use trigonometry properly. Three simple “words” to remember when to use one of the three functions (sin,cos,tan) is SOH CAH TOA which can be remember like this S(in, Opposite, Hypotenuse) C(os, Adjacent, Hypotenuse) T(an, Opposite, Adjacent). Below I have given an example of a simple problem, but with one of the less straightforward calculator steps (finding an angle). It isn’t a word problem but its the same thing when you look at the overall picture a word problem just gives you the information in a paragraph you have to find it yourself and plug it in, same thing here but you are given a filled out triangle: its basically just one step after the word problem (you would set this up).

In order to put other skills we learned this week to use (combining exponent laws) we need to know how to fully deal with the components within them, so I’m going to be explaining how to deal with integral exponents. Within expressions and equation’s you will need to know how to translate and transform negative exponents to simplify the equations/expressions further. Down below I showed how transforming the negative integers allows for simplifying the equation better.

Throughout this week we learned lessons, that built onto the new skills we learned the week before. I thought this one tied into my last weeks post of prime factorization well, because square roots remind me of simplifying of numbers, like used in prime factorization, you break the numbers down to help you solve the equation. One common problem with this process that can cause some confusion is that every mixed radical can be converted into an entire radical, while only some entire radicals can be expressed as a mixed number (depending if they have a factor that is a perfect square). There is an example of how you can convert these mixed radicals into entire ones.